
Sia

Question
Math
Posted 9 months ago
证明在以下两种立方晶格中单位体积交换能增量的公式:
筒立方:
面心立方: 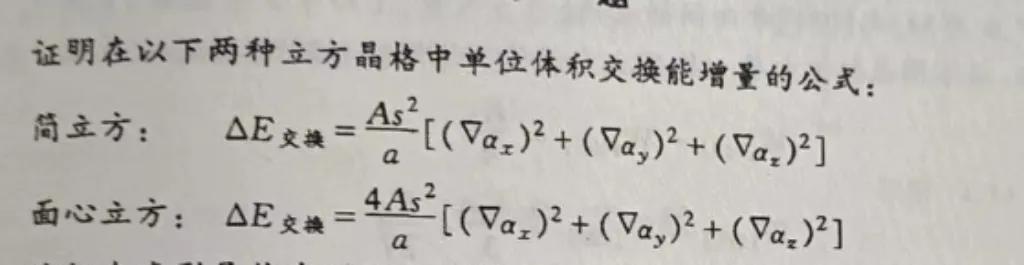

Answer from Sia
Posted 9 months ago
Solution by Steps
step 1
Identify the given equations for the two types of cubic lattices
step 2
For the simple cubic lattice, the formula is given by:
step 3
For the face-centered cubic lattice, the formula is given by:
step 4
Note the difference in the coefficients between the two formulas. For the simple cubic lattice, the coefficient is , while for the face-centered cubic lattice, the coefficient is
Answer
The formulas for the unit volume exchange energy increment in simple cubic and face-centered cubic lattices are correctly identified and differentiated by their coefficients.
Key Concept
Unit Volume Exchange Energy Increment
Explanation
The unit volume exchange energy increment formulas for simple cubic and face-centered cubic lattices differ by a factor of 4 in their coefficients, reflecting the different structural properties of the lattices.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question