
Sia
18. A projectile is fired at above the horizontal line with an initial velocity . At which of the following angles the projectile will land at the same distance as it is landed in the first trial?
A.
B.
C.
D. 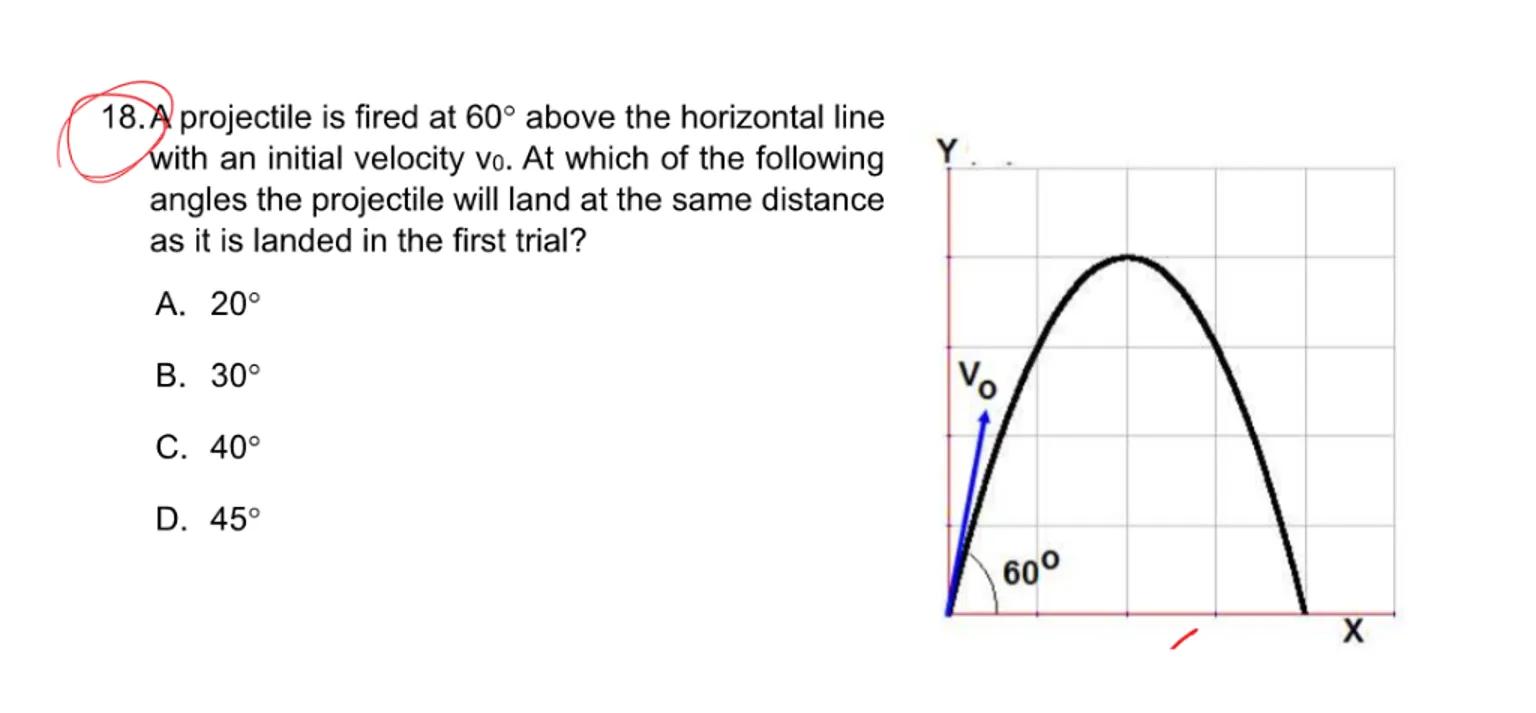

Answer from Sia
Posted about 1 year ago
Solution
a
The range of a projectile is given by the formula: where is the range, is the initial velocity, is the launch angle, and is the acceleration due to gravity. For the first trial, the angle is , so we calculate the range as: . Since $
b
To find another angle that gives the same range, we need to find an angle such that: . The angles that satisfy this equation are: for integer . This gives us: . Thus, one possible angle is
c
The other angle that can give the same range is . However, we also need to check the other options provided. The angle does not satisfy the equation, and neither do or . Therefore, the only angle that will land at the same distance as the first trial is
Answer
B
Key Concept
Projectile Motion: The range of a projectile is determined by its initial velocity and launch angle. The formula for range is , where is the acceleration due to gravity. The angles that yield the same range are complementary angles.
Explanation
The projectile launched at has a complementary angle of that will yield the same range, as both angles satisfy the sine function relationship for projectile motion.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question
