
Sia
第三问不对
4. (Probability Theory) An integrated circuit manufacturer tests the propagation delays of all chips of one particular batch. He discovers that the pdf of the delays is well approximated by a triangular distribution with mean value 8 ns , maximum value 12 ns and minimum value 4 ns . Find: (a) the variance of this distribution; (b) the standard deviation of the distribution; and (c) the percentage of chips which will be rejected if the specification for the device is 10 ns. 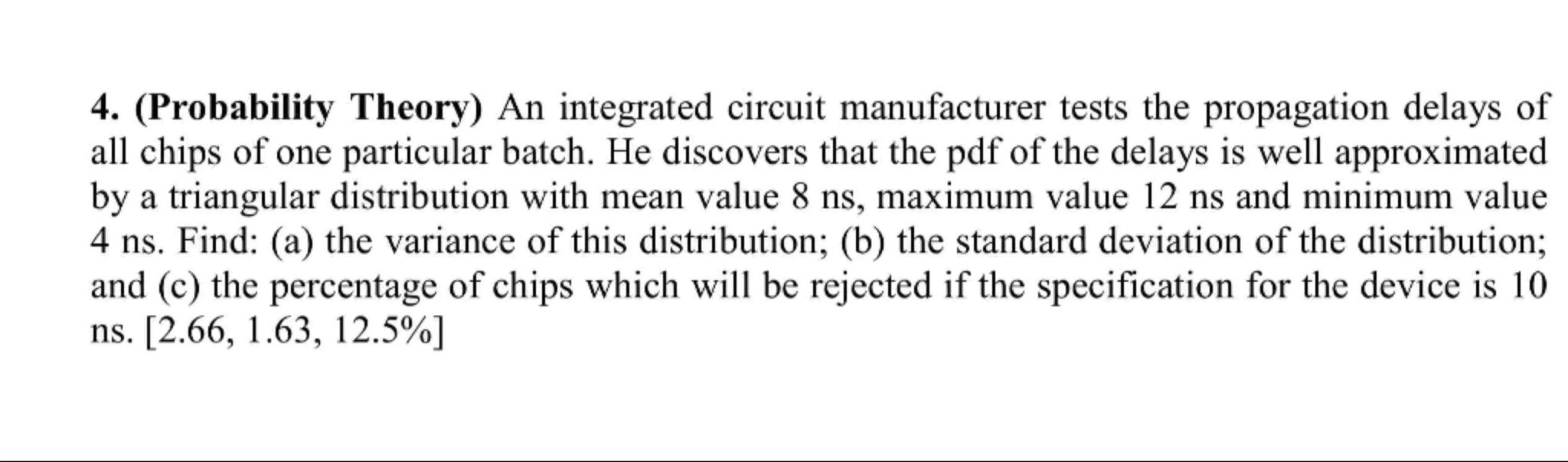

Answer from Sia
Posted about 1 year ago
你好,璐!我们来解决这个关于三角分布的问题。
给定的三角分布的参数是:
- 最小值
- 最大值
- 平均值
(a) 三角分布的方差公式为:
代入数值:
(b) 标准差是方差的平方根:
(c) 要计算被拒绝的芯片百分比,我们需要找出延迟大于 10 ns 的概率。对于三角分布,超过最大值的概率为 0,因此我们只需计算在 10 ns 处的累积分布函数值。
累积分布函数在 的值为:
因此,超过 10 ns 的概率为:
这意味着被拒绝的芯片百分比为:
所以,答案是:
(a) 2.67, (b) 1.63, (c) 43.75\%。
如果你还有其他问题,随时问我!Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question
