
Sia
6) At , cyclopropane rearranges to propene . The reaction is 1st order with a rate constant .
a. Write the rate equation, and determine the rate of reaction when the conversion is 0 and 0.5 , given that the initial concentration of
b. Integrate the rate equation and determine:
i. The concentration of cyclopropane after 30 minutes
ii. The time taken for the concentration of cyclopropane to fall to .
iii. The fractional conversion of cyclopropane and the concentration of propene after 50 minutes.
[0.866; 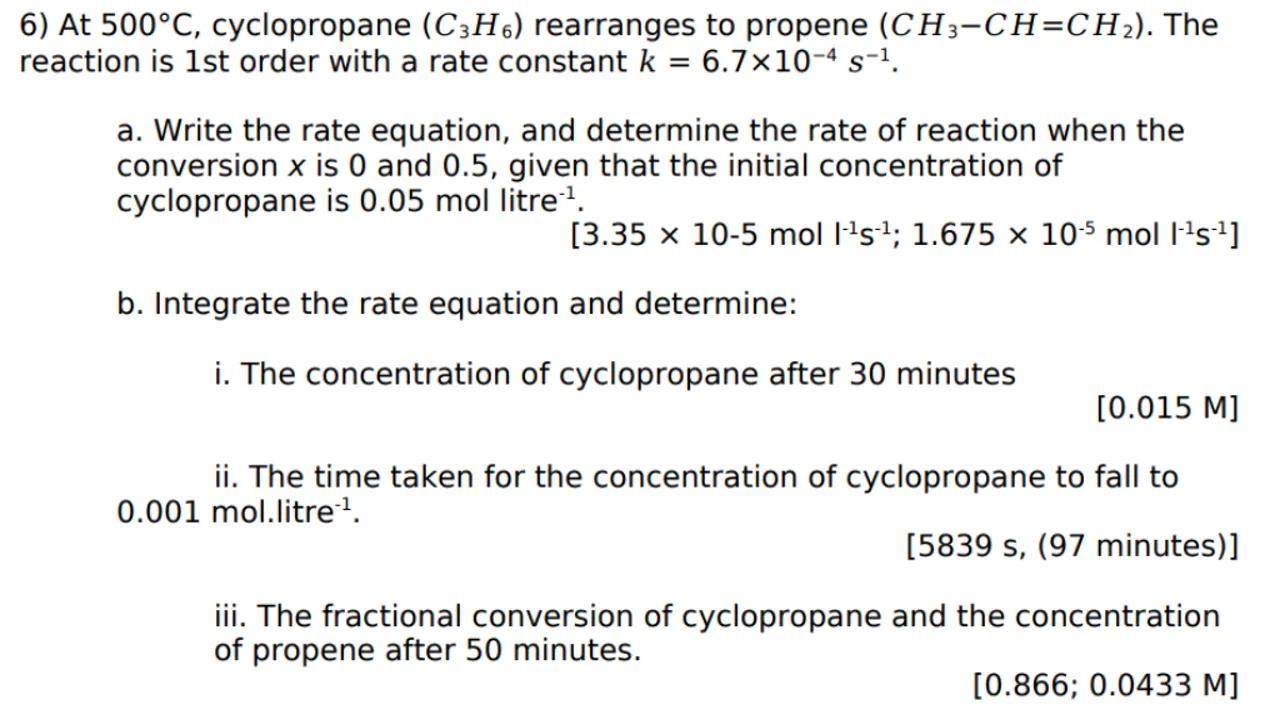

Answer from Sia
Posted over 1 year ago
Solution
1
Write the rate equation: For a first-order reaction, the rate equation is given by the formula , where is the rate constant and is the concentration of the reactant
2
Determine the rate of reaction at conversion $x = 0$: When , the concentration of cyclopropane is at its initial value. The rate of reaction is then
3
Determine the rate of reaction at conversion $x = 0.5$: When , the concentration of cyclopropane is half of its initial value. The rate of reaction is then
4
Integrate the rate equation to find concentration after 30 minutes: The integrated rate equation for a first-order reaction is . Solving for after 30 minutes (1800 seconds) gives the concentration of cyclopropane
5
Determine the time for concentration to fall to $0.001 \text{ M}$: Using the integrated rate equation, solve for when
6
Calculate the fractional conversion and concentration of propene after 50 minutes: The fractional conversion is given by . The concentration of propene is equal to the initial concentration of cyclopropane minus its concentration after 50 minutes
1 Answer
The rate of reaction at conversion is and at conversion is .
2 Answer
The concentration of cyclopropane after 30 minutes is .
3 Answer
The time taken for the concentration of cyclopropane to fall to is 5839 seconds (97 minutes).
4 Answer
The fractional conversion of cyclopropane after 50 minutes is 0.866, and the concentration of propene is .
Key Concept
The rate equation for a first-order reaction relates the rate of reaction to the concentration of the reactant and the rate constant.
Explanation
The rate of a first-order reaction decreases as the concentration of the reactant decreases, which is reflected in the rate of reaction at different conversion levels.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question
