
Sia

Question
Math
Posted over 1 year ago
A water tank holds 375 gallons. A drain is opened to let water out of the tank. The drain is closed 60 minutes later. The graph below shows the rate at which water drains from the tank as a function of time.
How much water remains in the tank after the drain is closed?
gallons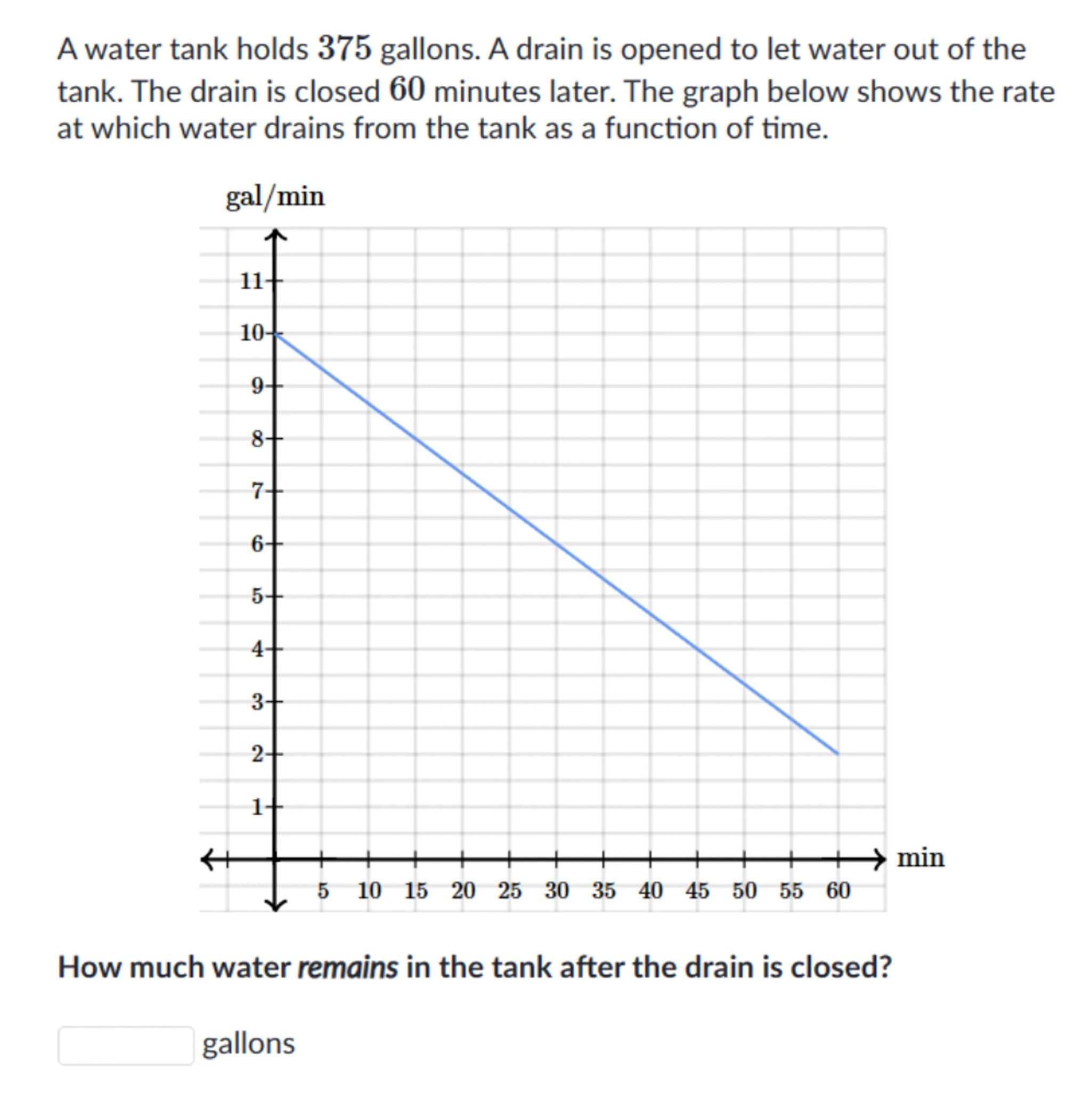

Answer from Sia
Posted over 1 year ago
Solution by Steps
step 1
The rate of water drainage is given by the function on the graph, which starts at 10 gal/min at and decreases linearly to 2 gal/min at minutes
step 2
To find the total amount of water drained, we need to integrate the rate function over the interval from to
step 3
The rate function can be approximated as a linear function:
step 4
Integrate from to :
step 5
Compute the integral:
step 6
Evaluate the definite integrals:
step 7
Subtract the total drained water from the initial amount:
Answer
15 gallons
Key Concept
Integration of a linear rate function
Explanation
To find the remaining water in the tank, we integrated the rate of drainage over the given time interval and subtracted the total drained water from the initial amount.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question