
Sia

Question
Math
Posted over 1 year ago
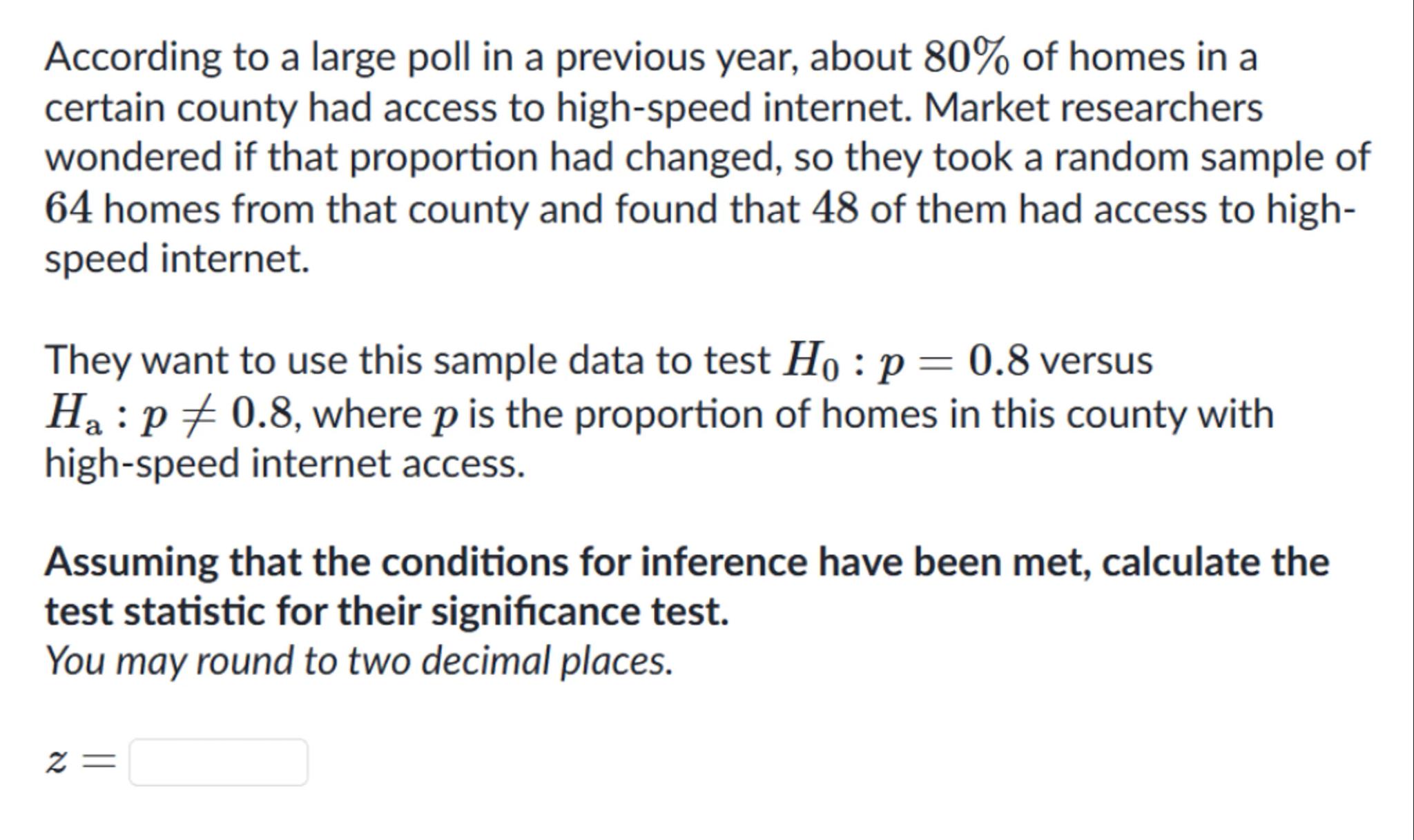
According to a large poll in a previous year, about of homes in a certain county had access to high-speed internet. Market researchers wondered if that proportion had changed, so they took a random sample of 64 homes from that county and found that 48 of them had access to highspeed internet.
They want to use this sample data to test versus , where is the proportion of homes in this county with high-speed internet access.
Assuming that the conditions for inference have been met, calculate the test statistic for their significance test.
You may round to two decimal places.

Answer from Sia
Posted over 1 year ago
Solution by Steps
step 1
Calculate the sample proportion by dividing the number of homes with high-speed internet by the total number of homes in the sample
$\hat{p} = \frac{48}{64}$
step 2
Compute the standard error (SE) using the null hypothesis proportion and the sample size
$SE = \sqrt{\frac{p_0(1 - p_0)}{n}}$ where $p_0 = 0.8$ and $n = 64$.
step 3
Calculate the z-test statistic using the sample proportion , the null hypothesis proportion , and the standard error SE
$z = \frac{\hat{p} - p_0}{SE}$
step 4
Substitute the values into the z-test statistic formula and calculate
$z = \frac{\frac{48}{64} - 0.8}{\sqrt{\frac{0.8(1 - 0.8)}{64}}}$
step 5
Simplify the calculations to find the z-test statistic
$z = \frac{0.75 - 0.8}{\sqrt{\frac{0.8 \cdot 0.2}{64}}}$
$z = \frac{-0.05}{\sqrt{\frac{0.16}{64}}}$
$z = \frac{-0.05}{0.05}$
$z = -1$
Answer
The test statistic for their significance test is .
Key Concept
Hypothesis Testing and Z-Test Statistic
Explanation
The z-test statistic is used in hypothesis testing to determine how many standard errors a sample proportion is from the hypothesized population proportion under the null hypothesis. It is calculated by subtracting the null hypothesis proportion from the sample proportion, then dividing by the standard error of the sample proportion.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question