
Sia

Question
Math
Posted over 1 year ago
An airline transports over 10,000 passengers daily, and the airline is curious what proportion of their passengers use mobile boarding passes instead of paper passes. They take an SRS of 80 passengers and find that 60 of them use mobile boarding passes.
Based on this sample, which of the following is a confidence interval for the proportion of passengers who use mobile boarding passes?
Choose 1 answer:
(A)
(B)
(c)
(D) 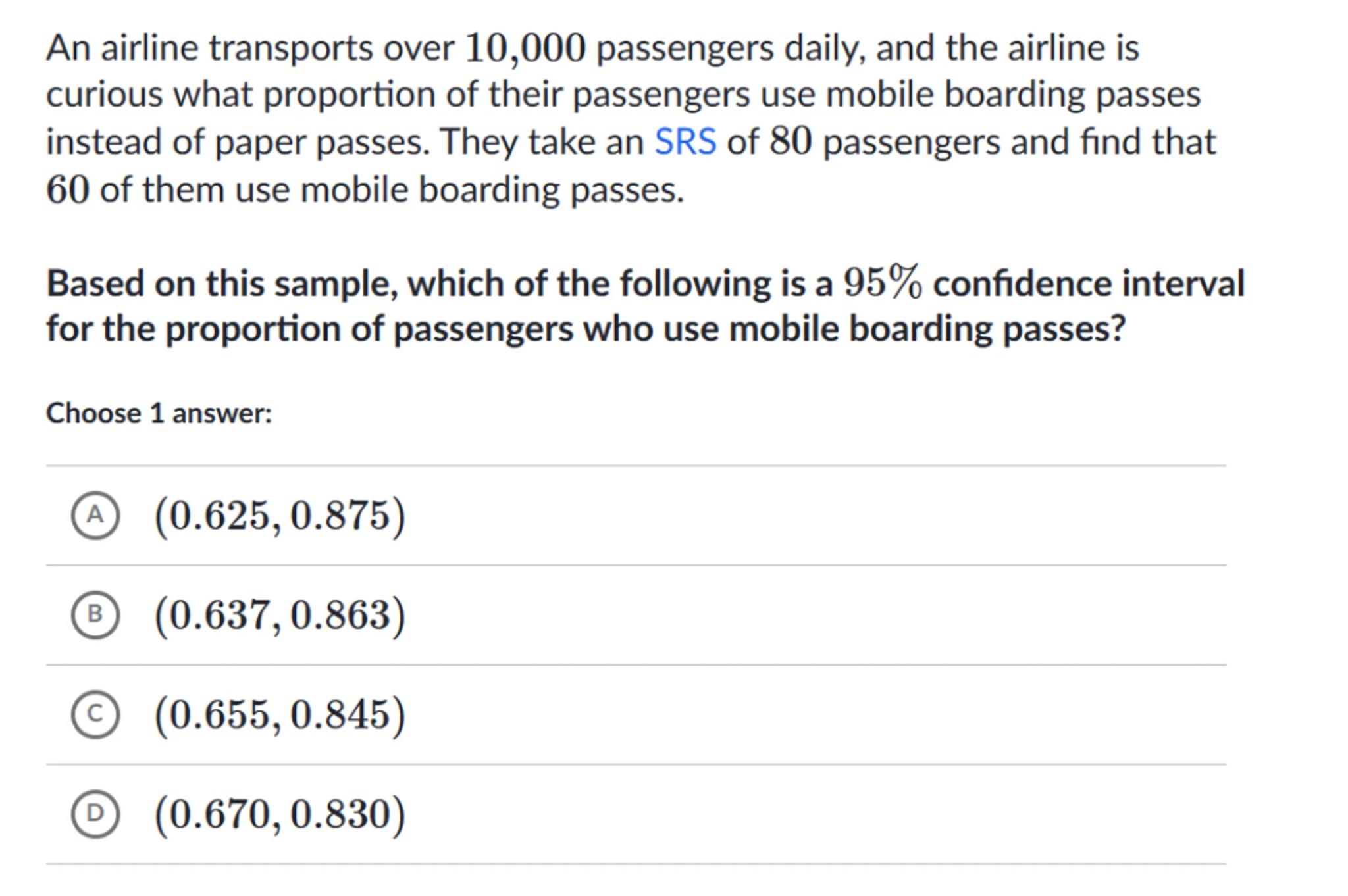

Answer from Sia
Posted over 1 year ago
Solution by Steps
step 2
Find the standard error (SE) of the sample proportion using the formula , where is the sample size:
step 3
Calculate the value of the standard error:
step 4
Determine the z-score for a 95% confidence interval, which is approximately 1.96 for a two-tailed test
step 5
Calculate the margin of error (ME) using the z-score and the standard error:
step 6
Find the lower and upper bounds of the 95% confidence interval by subtracting and adding the margin of error to the sample proportion: lower bound and upper bound
step 7
Round the lower and upper bounds to three decimal places: lower bound and upper bound
C
Key Concept
Confidence Interval for a Proportion
Explanation
The confidence interval for a proportion is calculated using the sample proportion, the z-score corresponding to the desired confidence level, and the standard error of the sample proportion. It provides a range of values within which the true population proportion is likely to fall.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question