
Sia

Question
Math
Posted over 1 year ago
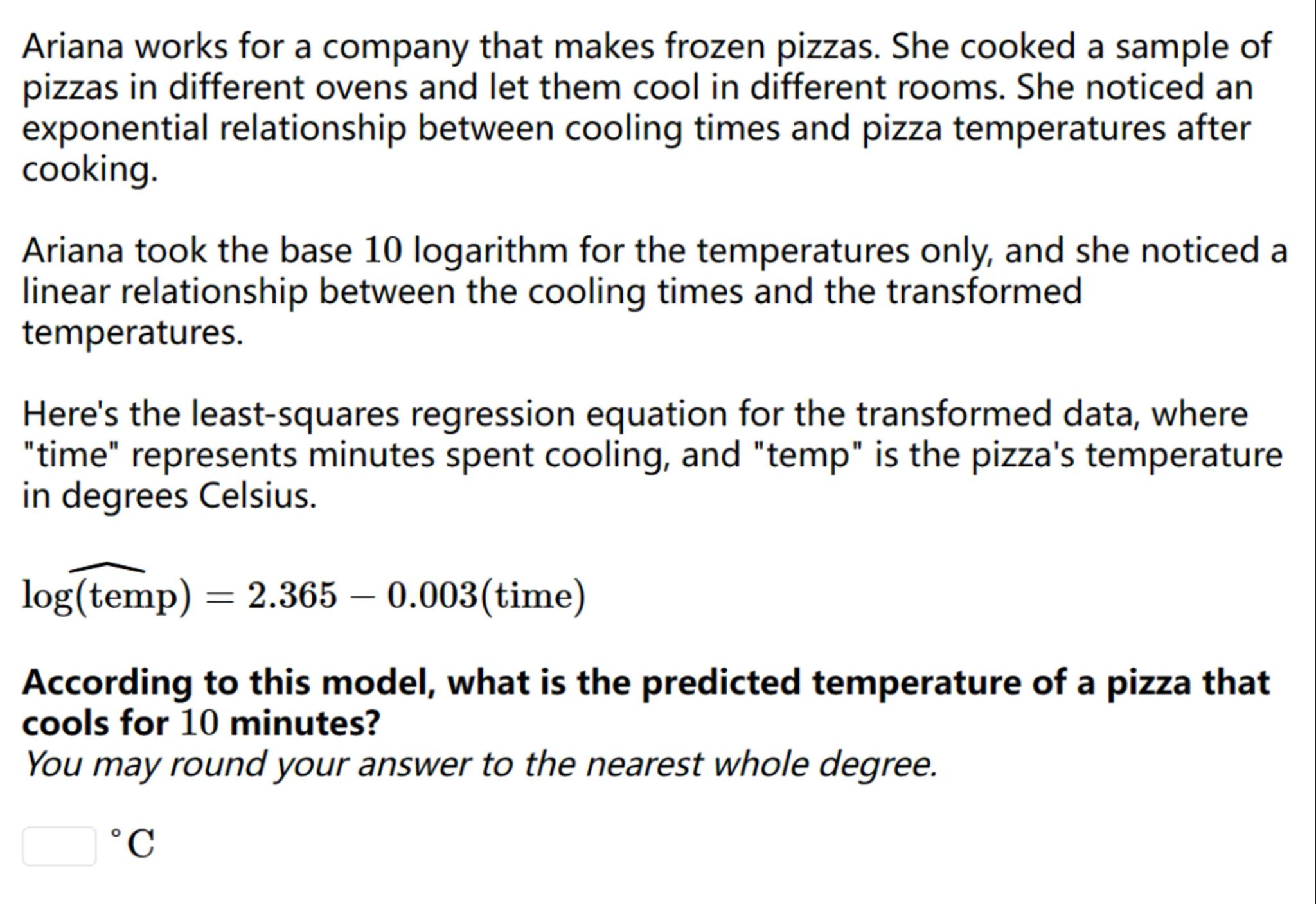
Ariana works for a company that makes frozen pizzas. She cooked a sample of pizzas in different ovens and let them cool in different rooms. She noticed an exponential relationship between cooling times and pizza temperatures after cooking.
Ariana took the base 10 logarithm for the temperatures only, and she noticed a linear relationship between the cooling times and the transformed temperatures.
Here's the least-squares regression equation for the transformed data, where "time" represents minutes spent cooling, and "temp" is the pizza's temperature in degrees Celsius.
According to this model, what is the predicted temperature of a pizza that cools for 10 minutes?
You may round your answer to the nearest whole degree.

Answer from Sia
Posted over 1 year ago
Solution by Steps
step 1
To find the predicted temperature after 10 minutes, we first calculate the value of the logarithm of the temperature using the regression equation
step 2
Substitute the time value into the regression equation:
step 3
Perform the calculation:
step 4
According to the asksia-ll calculation list, the result is
step 5
To find the temperature, we need to take the antilog (base 10) of the logarithmic result:
step 6
Using the asksia-ll calculator result, the antilog of 2.335 is approximately 216.272
step 7
Round the temperature to the nearest whole degree:
Answer
The predicted temperature of a pizza that cools for 10 minutes is approximately 216 degrees Celsius.
Key Concept
Logarithmic to Exponential Conversion
Explanation
To find the temperature from the logarithmic form, we convert the log equation back to its exponential form using the antilogarithm (base 10).
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question