
Sia

Question
Math
Posted over 1 year ago
Assume that is a negatively oriented, simple, closed curve. Let be the region enclosed by .
Use the normal form of Green's theorem to rewrite as a double integral.
Choose 1 answer:
(A)
(B)
(C)
(D)
() Green's theorem is not necessarily applicable.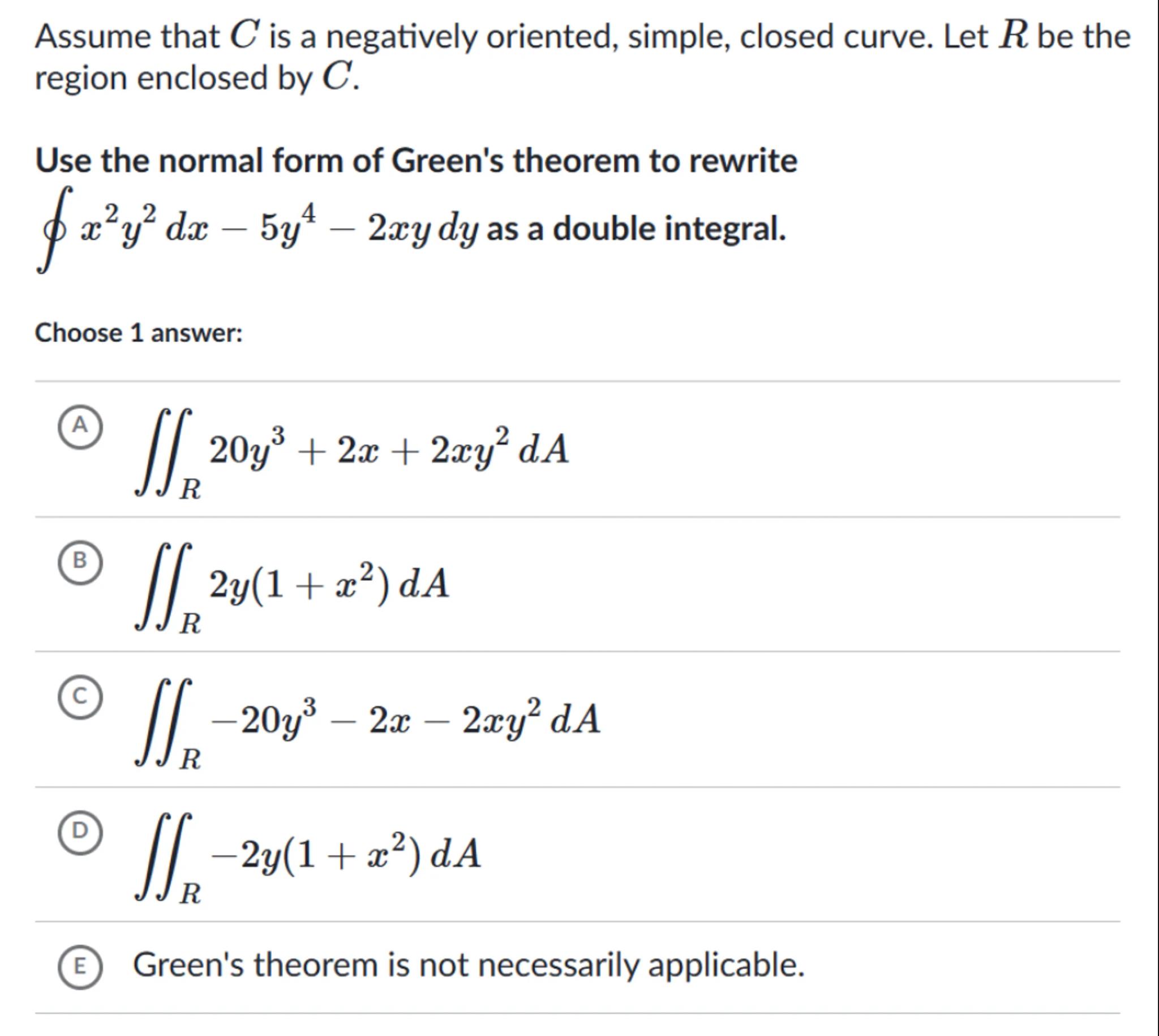

Answer from Sia
Posted over 1 year ago
Solution by Steps
step 2
Identify and from the integral. Here, and
step 3
Compute the partial derivatives and . We find and
step 4
Substitute the partial derivatives into the formula from Green's theorem. We get
step 5
Simplify the double integral expression. The integral becomes
step 6
Since is negatively oriented, we need to take the negative of the integral we found to correct the orientation. This gives us
[question number] Answer
B
Key Concept
Green's theorem and orientation of curve
Explanation
Green's theorem relates a line integral around a simple, closed curve to a double integral over the region it encloses. The orientation of the curve is important; if the curve is negatively oriented, the result of Green's theorem must be negated to match the orientation.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question