
Sia

Question
Math
Posted over 1 year ago
Consider the following integral:
(a) Locate and characterize all singularities of the integrand in the complex plane.
(b) Locate and characterize all singularities of the integrand when is replaced by (and by the complex variable ), i.e. .
(c) Define the contours required to evaluate the given integral .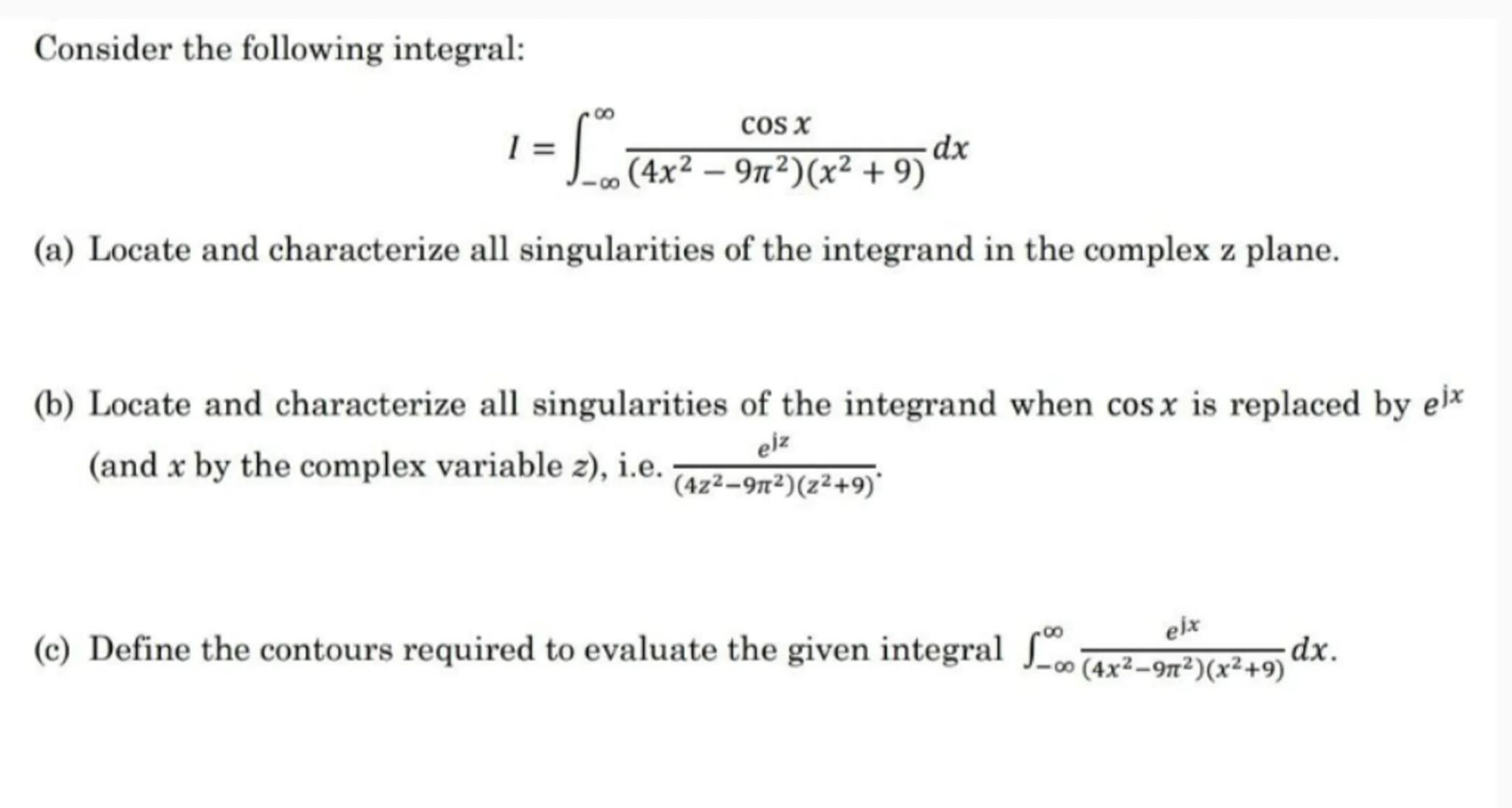

Answer from Sia
Posted over 1 year ago
Solution by Steps
step 1
Identify the integrand:
step 2
Set the denominators to zero to find singularities: and
step 3
Solve :
step 4
Solve :
step 5
The singularities are (real) and (imaginary)
(b) Locate and characterize all singularities of the integrand when is replaced by (and by the complex variable ).
step 1
Identify the new integrand:
step 2
The singularities remain the same as in part (a): and
step 3
Characterize the singularities: They are simple poles
(c) Define the contours required to evaluate the given integral .
step 1
Use the residue theorem to evaluate the integral
step 2
Choose a semicircular contour in the upper half-plane to avoid the poles on the real axis
step 3
The contour should enclose the poles at and
step 4
Evaluate the residues at these poles to find the integral
Answer
The singularities of the integrand are and . When is replaced by , the singularities remain the same and are simple poles. To evaluate the integral, use a semicircular contour in the upper half-plane that encloses the poles at and .
Key Concept
Singularities and Contour Integration
Explanation
The key concept involves identifying singularities of the integrand and using contour integration to evaluate the integral. The singularities are found by setting the denominator to zero, and the contour is chosen to apply the residue theorem effectively.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question