Asksia AI LOGO

Sia

Question
Math
Posted 7 months ago

Consider the following two prisms.

Sandra tried to use Cavalieri's principle to show that the two figures have the same volume.
"The base areas are the same. Therefore, corresponding crosssections have the same area. The prisms have the same height. So the volumes must be the same."

What is the first mistake Sandra made?

Choose 1 answer:
(A) The base areas are not the same.
(B) It isn't true that corresponding cross-sections have the same area.
(C) The heights are not the same.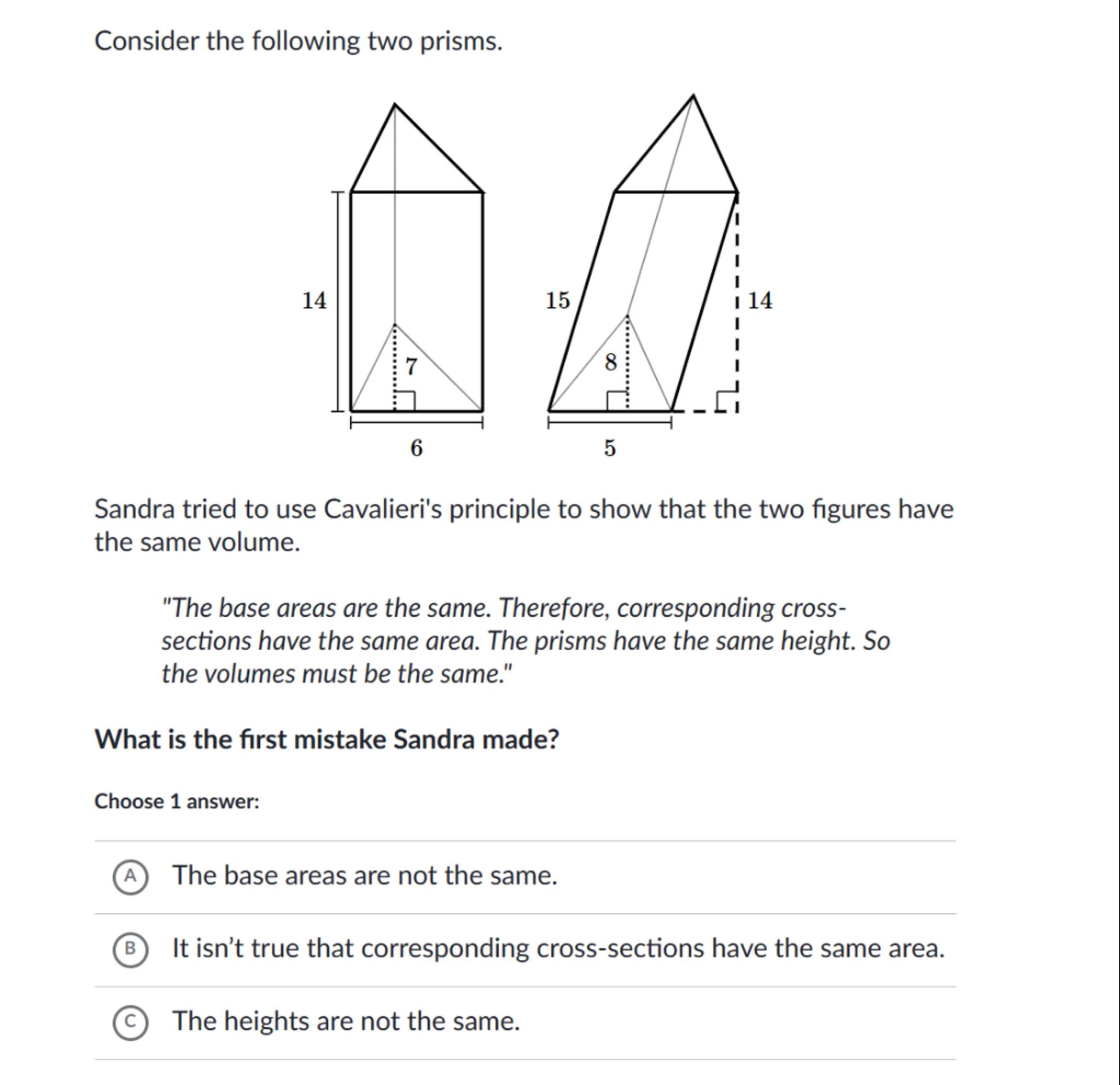
Sign in to unlock the answer
Answer from Sia
Posted 7 months ago
Solution by Steps
step 2
The volume of the first prism with base 6, height 7, and length 14 is calculated as: V1=12⋅base⋅height⋅length=12⋅6⋅7⋅14=294V_1 = \frac{1}{2} \cdot \text{base} \cdot \text{height} \cdot \text{length} = \frac{1}{2} \cdot 6 \cdot 7 \cdot 14 = 294
step 3
The volume of the second prism with base 5, height 8, and length 15 is calculated as: V2=12⋅base⋅height⋅length=12⋅5⋅8⋅15=300V_2 = \frac{1}{2} \cdot \text{base} \cdot \text{height} \cdot \text{length} = \frac{1}{2} \cdot 5 \cdot 8 \cdot 15 = 300
step 4
Compare the volumes of the two prisms to identify the first mistake Sandra made
[question number] Answer
A
Key Concept
Cavalieri's Principle and Volume Calculation
Explanation
Cavalieri's Principle states that if two solids have the same height and the same cross-sectional area at every level, then they have the same volume. Sandra's first mistake was assuming that the base areas of the two prisms are the same, which is not true as shown by the different volumes calculated.

Not the question you are looking for? Ask here!

Enter question by text

Enter question by image

Unlock Smarter Learning with AskSia Super!

Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.

30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question