
Sia

Question
Math
Posted over 1 year ago
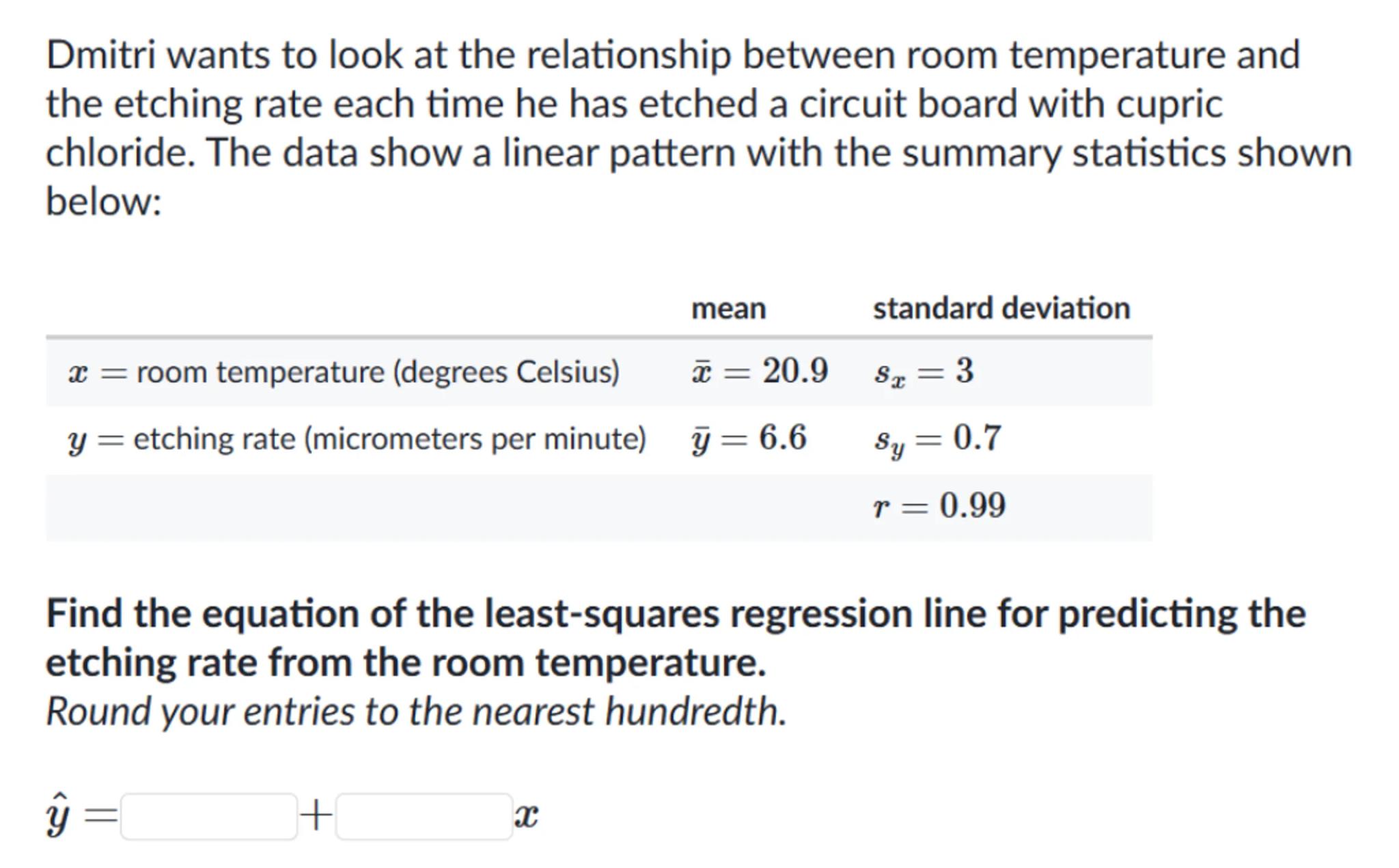
Dmitri wants to look at the relationship between room temperature and the etching rate each time he has etched a circuit board with cupric chloride. The data show a linear pattern with the summary statistics shown below:
\begin{tabular}{lll}
& mean & standard deviation \\
\hline room temperature (degrees Celsius) & & \\
etching rate (micrometers per minute) & & \\
& &
\end{tabular}
Find the equation of the least-squares regression line for predicting the etching rate from the room temperature.
Round your entries to the nearest hundredth.

Answer from Sia
Posted over 1 year ago
Solution by Steps
step 1
Calculate the slope (b) of the least-squares regression line using the formula
step 2
Substitute the given values , , and into the formula to find
step 3
Compute the slope:
step 4
Calculate the y-intercept (a) of the line using the formula
step 5
Substitute the mean values and and the calculated slope into the formula to find
step 6
Compute the y-intercept:
step 7
Round the values of and to the nearest hundredth to get the final equation of the least-squares regression line
Answer
The equation of the least-squares regression line is .
Key Concept
Least-Squares Regression Line
Explanation
The least-squares regression line is used to predict the value of a dependent variable based on the value of an independent variable. The line is determined by its slope and y-intercept, which can be calculated using the mean values, standard deviations, and correlation coefficient of the data.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question