
Sia
Exercise 8. Cyclobutane decomposes to ethylene by the following reaction:
Determine the order of the reaction and the rate constant on the basis of the pressure drift. These pressures were measured during the reaction at in a tank with constant volume.
order
\begin{tabular}{cc}
\hline Time & \\
\hline 0 & 400 \\
2000 & 316 \\
4000 & 248 \\
6000 & 196 \\
8000 & 155 \\
10000 & 122 \\
\hline
\end{tabular}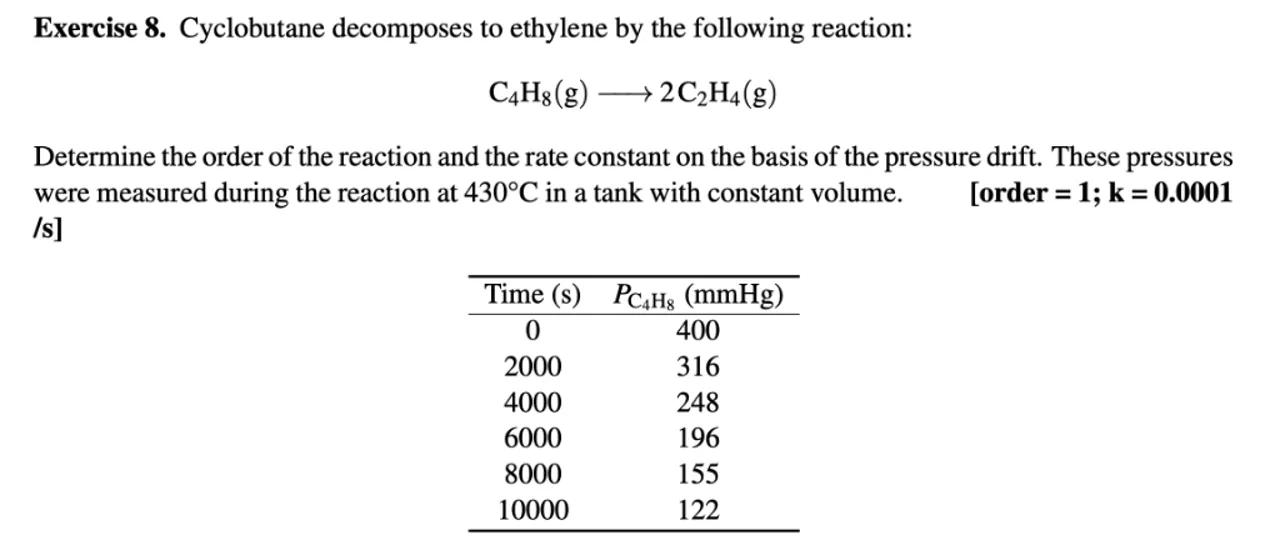

Answer from Sia
Posted over 1 year ago
Solution
1
Identify the reaction: The reaction given is the decomposition of cyclobutane () into ethylene (). The balanced chemical equation is:
2
Determine the order of the reaction: To determine the order of the reaction, we need to analyze the pressure data provided. The pressure of decreases over time, which suggests a first-order reaction. For a first-order reaction, the rate law is:
We can confirm this by plotting versus time and checking for a linear relationship
3
Calculate the rate constant (k): For a first-order reaction, the integrated rate law is:
Using the data provided, we can calculate by finding the slope of the line from the plot of versus time. The slope of this line is equal to . Given the data:
We can calculate for each time point and then determine the slope
4
Verify the order and rate constant: After plotting versus time, we should get a straight line if the reaction is first-order. The slope of this line will give us the rate constant . Given the provided answer, we can verify:
Answer
The reaction is first-order with a rate constant .
Key Concept
First-order reaction kinetics
Explanation
The decomposition of cyclobutane to ethylene follows first-order kinetics, as indicated by the linear relationship between and time. The rate constant is determined from the slope of this line.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question