
Sia
需要更详细的解题步骤
Exercise and Solution set 1_corrected(1).pdf
5. (Probability Theory, Fourier Transform) and are independent random signals which have the following pdfs:
Use the characteristics of Fourier transform to find the pdf of their sum.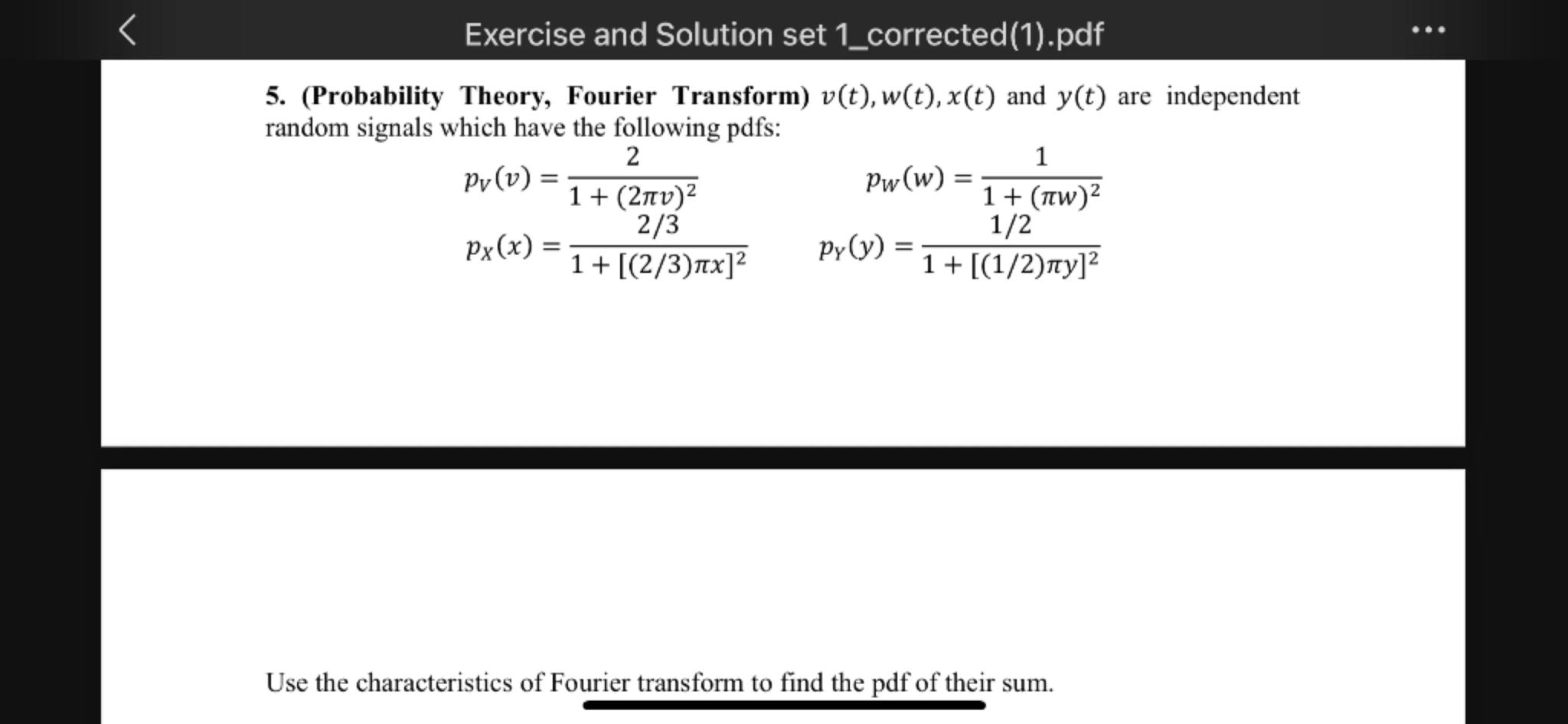

Answer from Sia
Posted about 1 year ago
你好,璐!我们来解决这个关于概率理论和傅里叶变换的问题。
给定的随机信号的概率密度函数(pdf)如下:
由于这些信号是独立的,我们可以使用傅里叶变换的特性来找到它们和的概率密度函数。根据傅里叶变换的性质,两个独立随机变量的和的概率密度函数是它们各自概率密度函数的傅里叶变换的乘积。
1. 首先,计算每个信号的傅里叶变换。
2. 然后,将这些傅里叶变换相乘。
3. 最后,对乘积进行逆傅里叶变换,以获得和的概率密度函数。
具体的计算步骤可能会涉及到一些复杂的积分和变换,但这是解决这个问题的基本思路。
如果你需要更详细的计算步骤或有其他问题,请告诉我!Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question
