
Sia
Find constants and such that the vector field defined by is irrotational. With these values of and , determine a scalar function such that .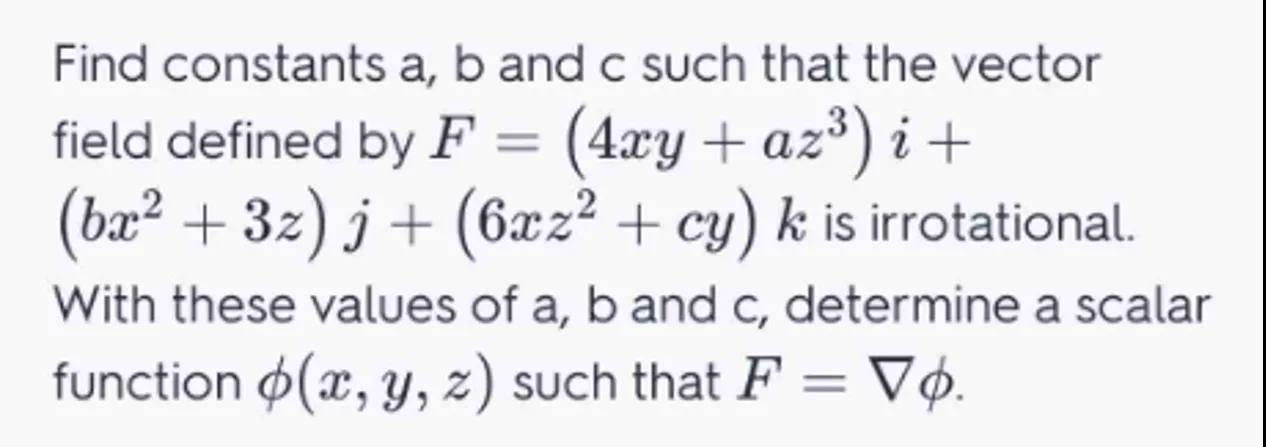

Answer from Sia
Posted over 1 year ago
Solution by Steps
step 1
To determine the constants , , and such that the vector field is irrotational, we need to ensure that the curl of is zero
step 2
The curl of is given by
step 3
Calculate :
step 4
Calculate :
step 5
For the component to be zero:
step 6
Calculate :
step 7
Calculate :
step 8
For the component to be zero:
step 9
Calculate :
step 10
Calculate :
step 11
For the component to be zero:
step 12
With , , and , we now find the scalar function such that
step 13
Integrate with respect to :
step 14
Differentiate with respect to and set it equal to :
step 15
Differentiate with respect to and set it equal to :
step 16
Therefore, the scalar function is
Answer
The constants are , , and . The scalar function is .
Key Concept
Irrotational Vector Field
Explanation
To find the constants that make a vector field irrotational, we set the curl of the vector field to zero and solve for the constants. Then, we find the scalar potential function by integrating the components of the vector field.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question