
Sia

Question
Math
Posted over 1 year ago
Find the perimeter of .
If entering your answer as a decimal, round your final answer to the nearest hundredth.
units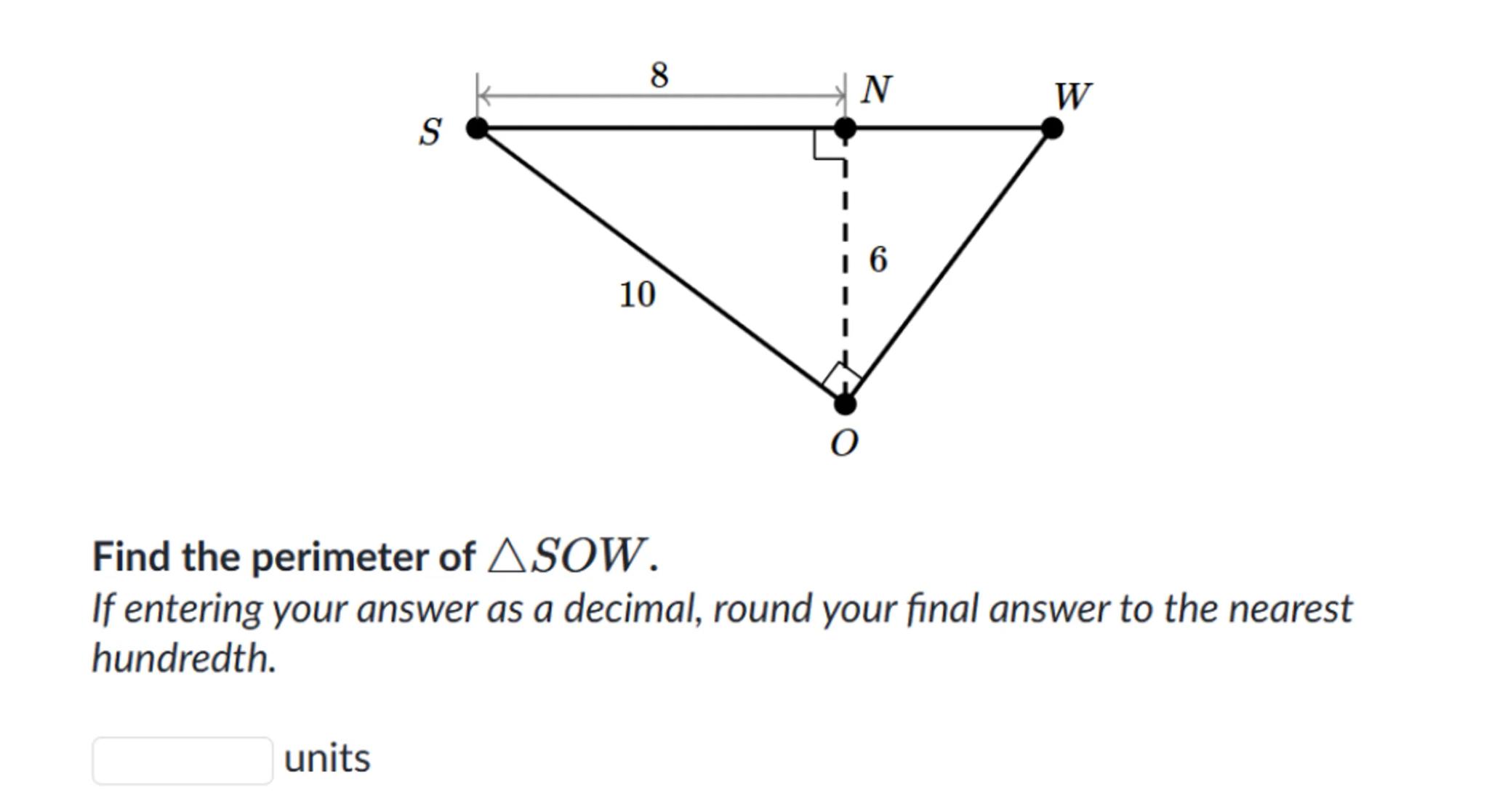

Answer from Sia
Posted over 1 year ago
Solution by Steps
step 1
Identify the lengths of the sides of the triangle ΔSWO
step 2
The lengths are given as SN = 8 units, SO = 10 units, and NO = 6 units
step 3
To find the perimeter of ΔSWO, add the lengths of the sides SN, SO, and the full length of SW
step 4
Since NO is a dashed line and there is a right angle where line NO meets line SW, we infer that ΔSNO is a right triangle
step 5
Use the Pythagorean theorem to find the length of SW:
step 6
Calculate SW: units
step 7
Now, add the lengths of SN, SO, and SW to find the perimeter:
step 8
Calculate the perimeter: units
[1] Answer
The perimeter of is 28 units.
Key Concept
Finding the perimeter of a triangle
Explanation
The perimeter of a triangle is the sum of the lengths of its sides. For right triangles, the Pythagorean theorem can be used to find the length of the hypotenuse if the lengths of the other two sides are known.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question