
Sia

Question
Math
Posted over 1 year ago
Function is graphed.
What appears to be the value of ?
Choose 1 answer:
(A) 2
(B) -2
(C) -6
(D) Unbounded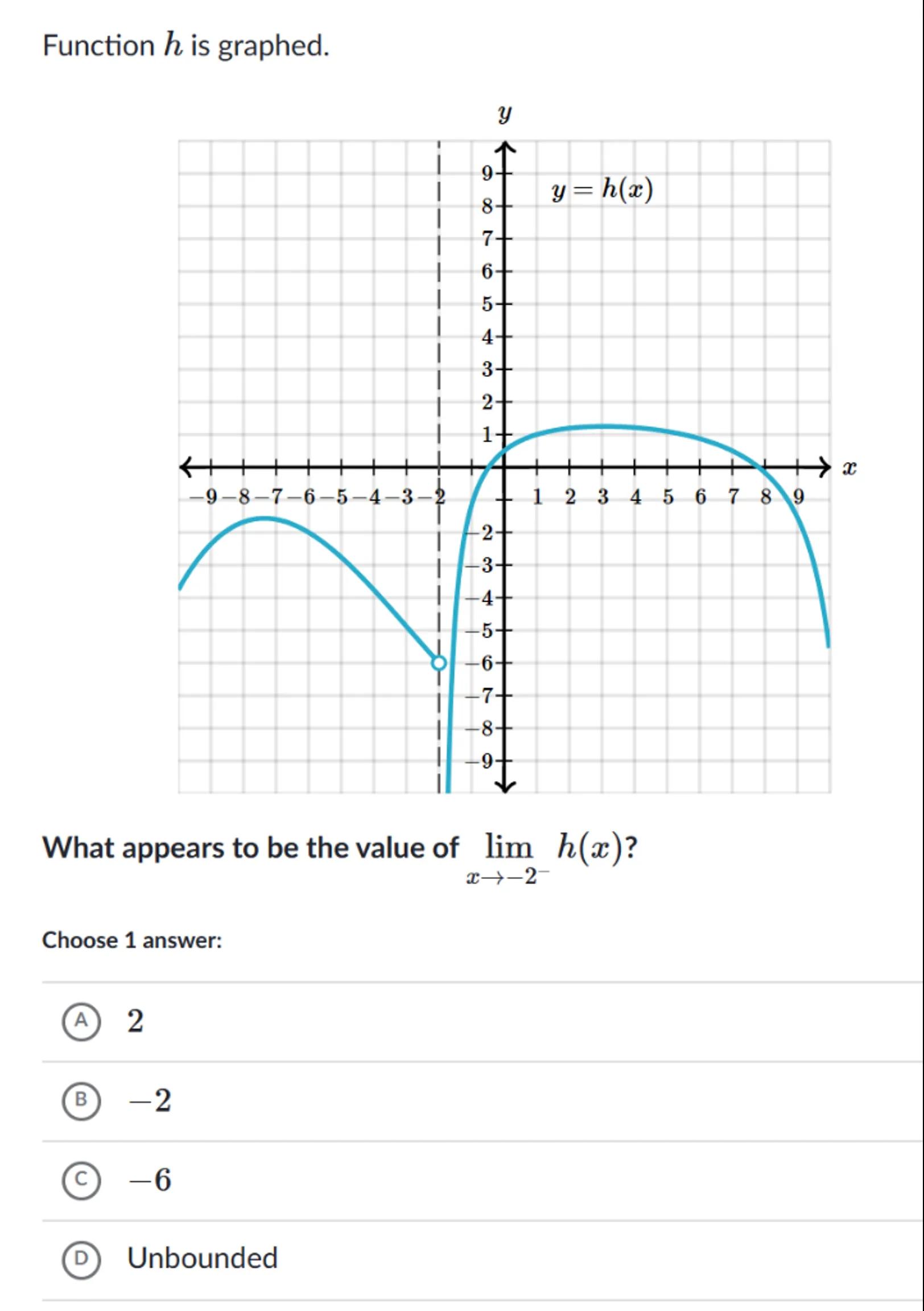

Answer from Sia
Posted over 1 year ago
Solution by Steps
step 1
To find the limit of as approaches from the left, we need to analyze the behavior of the function near
step 2
From the graph, we observe that as approaches from the left, approaches
step 3
Since the function approaches the same value from the left, the limit exists and is equal to
step 4
Therefore,
Answer
(C) -6
Key Concept
Limit of a function
Explanation
The limit of a function as approaches a certain value is the value that the function approaches as gets closer to that value. In this case, as approaches from the left, approaches .
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question