
Sia

Question
Math
Posted over 1 year ago
Here's a partially-filled Hessian matrix.
What is the missing entry?
Choose 1 answer:
(A)
(B)
(C)
(D) There's not enough information.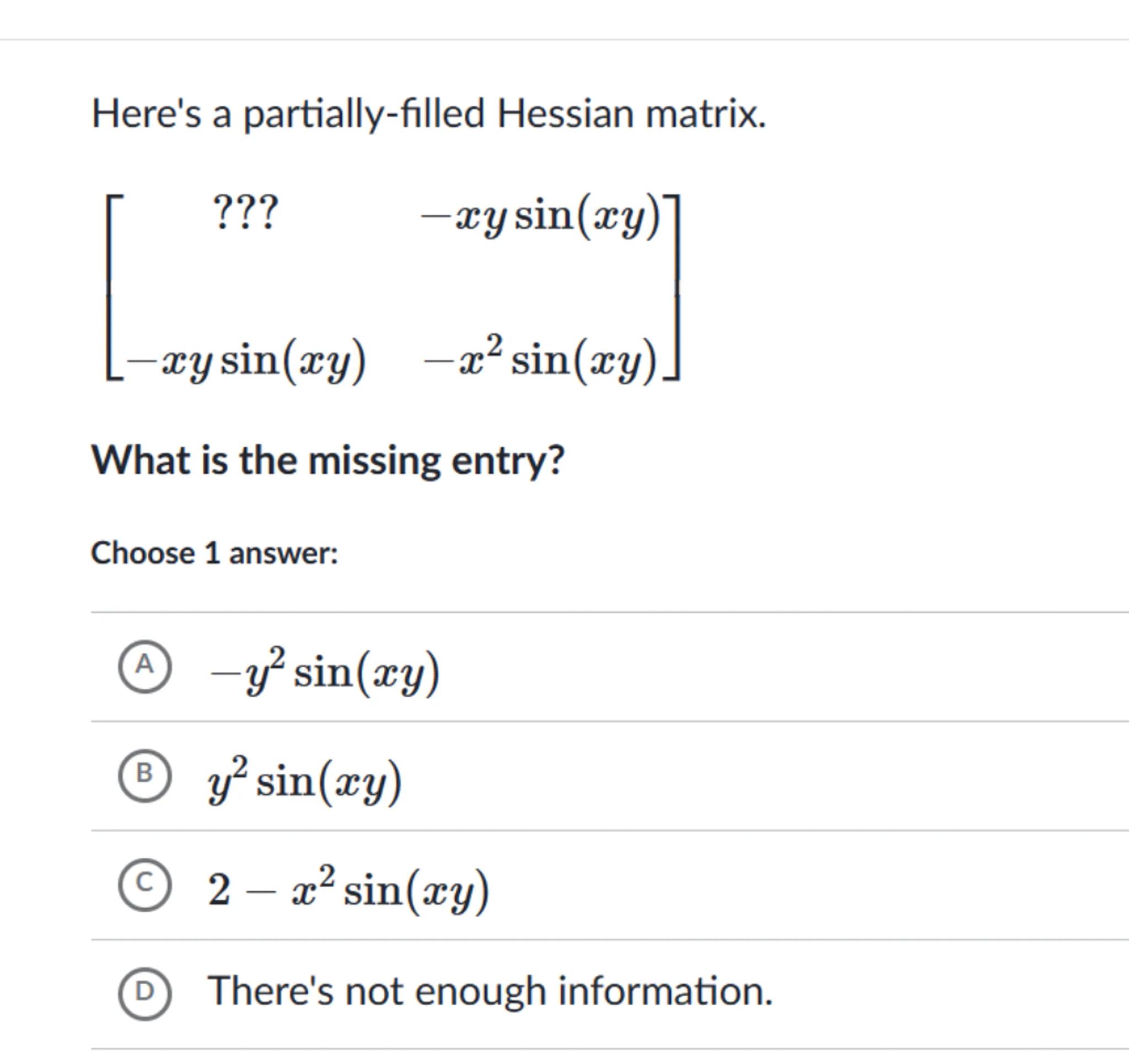

Answer from Sia
Posted over 1 year ago
Solution by Steps
step 2
The first partial derivative of the function with respect to x is
step 3
The second partial derivative of the function with respect to x is
step 4
Simplifying the second partial derivative with respect to x, we get
step 5
Combining like terms, the second partial derivative with respect to x is . This is the missing entry in the Hessian matrix
A
Key Concept
Hessian Matrix and Second Partial Derivatives
Explanation
The Hessian matrix is composed of second-order partial derivatives of a function. The diagonal entries are the second partial derivatives with respect to each variable, which in this case is x for the missing entry.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question