
Sia

Question
Math
Posted over 1 year ago
Holden tried to prove that .
\begin{tabular}{lll}
& Statement & Reason \\
\hline 1 & & Given \\
2 & & Line segments are congruent to themselves. \\
3 & & Side-side congruence
\end{tabular}
What is the first error Holden made in his proof?
Choose 1 answer:
(A) Holden used an invalid reason to justify the congruence of a pair of sides or angles.
(B) Holden only established some of the necessary conditions for a congruence criterion.
(c) Holden established all necessary conditions, but then used an inappropriate congruence criterion.
(D) Holden used a criterion that does not guarantee congruence.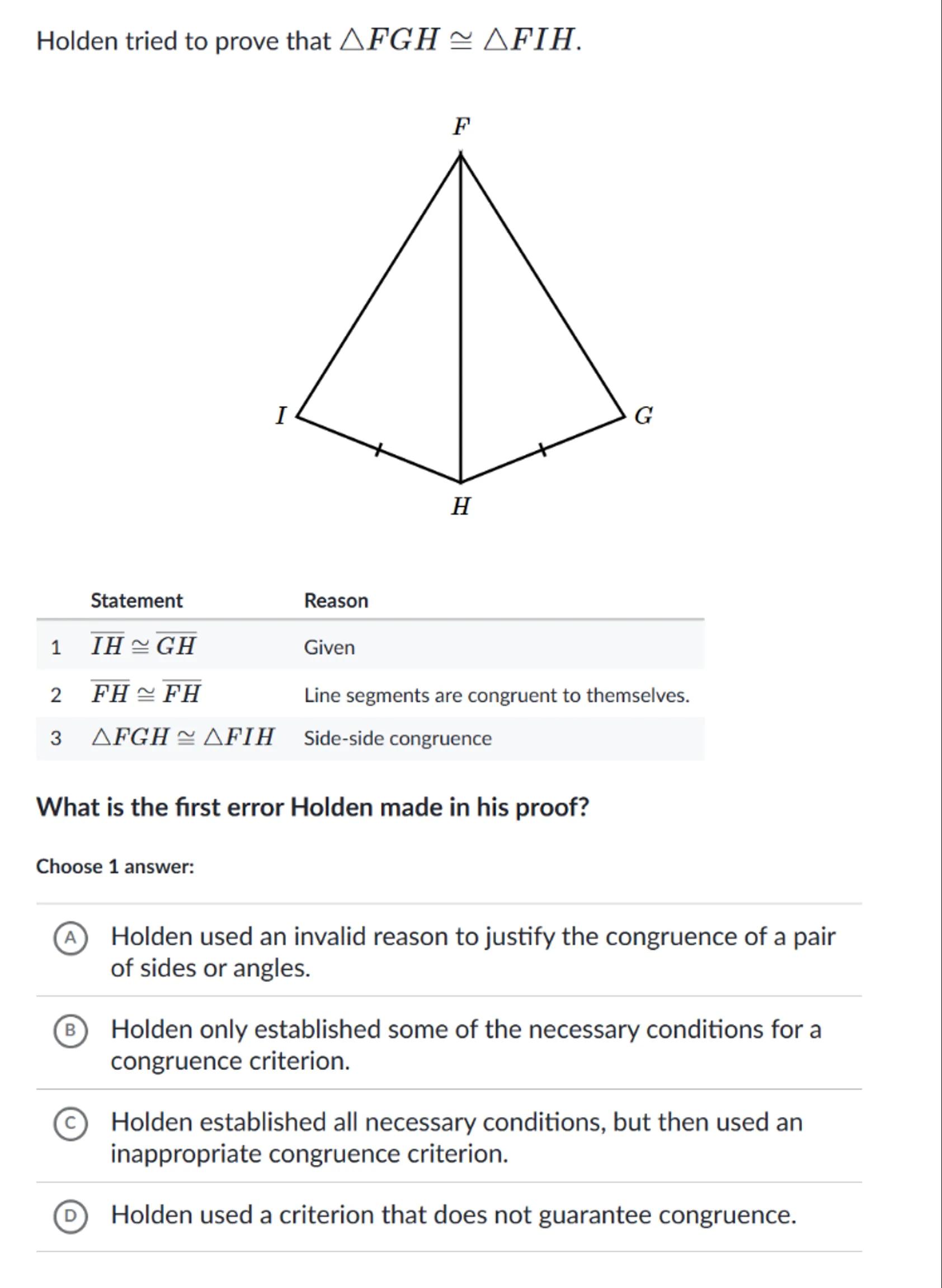

Answer from Sia
Posted over 1 year ago
Solution by Steps
step 2
The first statement is that , with the reason given as "Given". This is a valid statement and reason
step 3
The second statement is that , with the reason "Line segments are congruent to themselves". This is also a valid statement and reason
step 4
The third statement claims that based on "Side-side congruence". However, there is no such congruence criterion as "Side-side congruence". The correct criteria are Side-Side-Side (SSS), Side-Angle-Side (SAS), Angle-Side-Angle (ASA), Angle-Angle-Side (AAS), and Hypotenuse-Leg (HL) for right triangles
step 5
Since Holden has only shown two sides to be congruent and has not established the congruence of the third side or any angles, he has not satisfied any of the valid congruence criteria
1 Answer
D
Key Concept
Triangle Congruence Criteria
Explanation
To prove two triangles congruent, one must satisfy one of the valid congruence criteria: SSS, SAS, ASA, AAS, or HL for right triangles. "Side-side congruence" is not a recognized criterion.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question