
Sia

Question
Math
Posted over 1 year ago
Jerry took a sample of 4 employees in his office and observed how many hours they each worked one day. Here is what he found:
\begin{tabular}{lrrrrr}
Employee & Leslie & April & Tom & Andy \\
\cline { 1 - 2 } \cline { 3 - 4 } & 10 & 2 & 4 & 8
\end{tabular}
Jerry found their mean was hours. He thinks the standard deviation is
What is the error in Jerry's standard deviation calculation?
Choose 1 answer:
(A) He shouldn't take the square root at all.
(B) He should only take the square root of the numerator.
(c) His denominator is incorrect.
(D) He shouldn't square each deviation in the numerator.
(E) There is no error; his calculation is correct.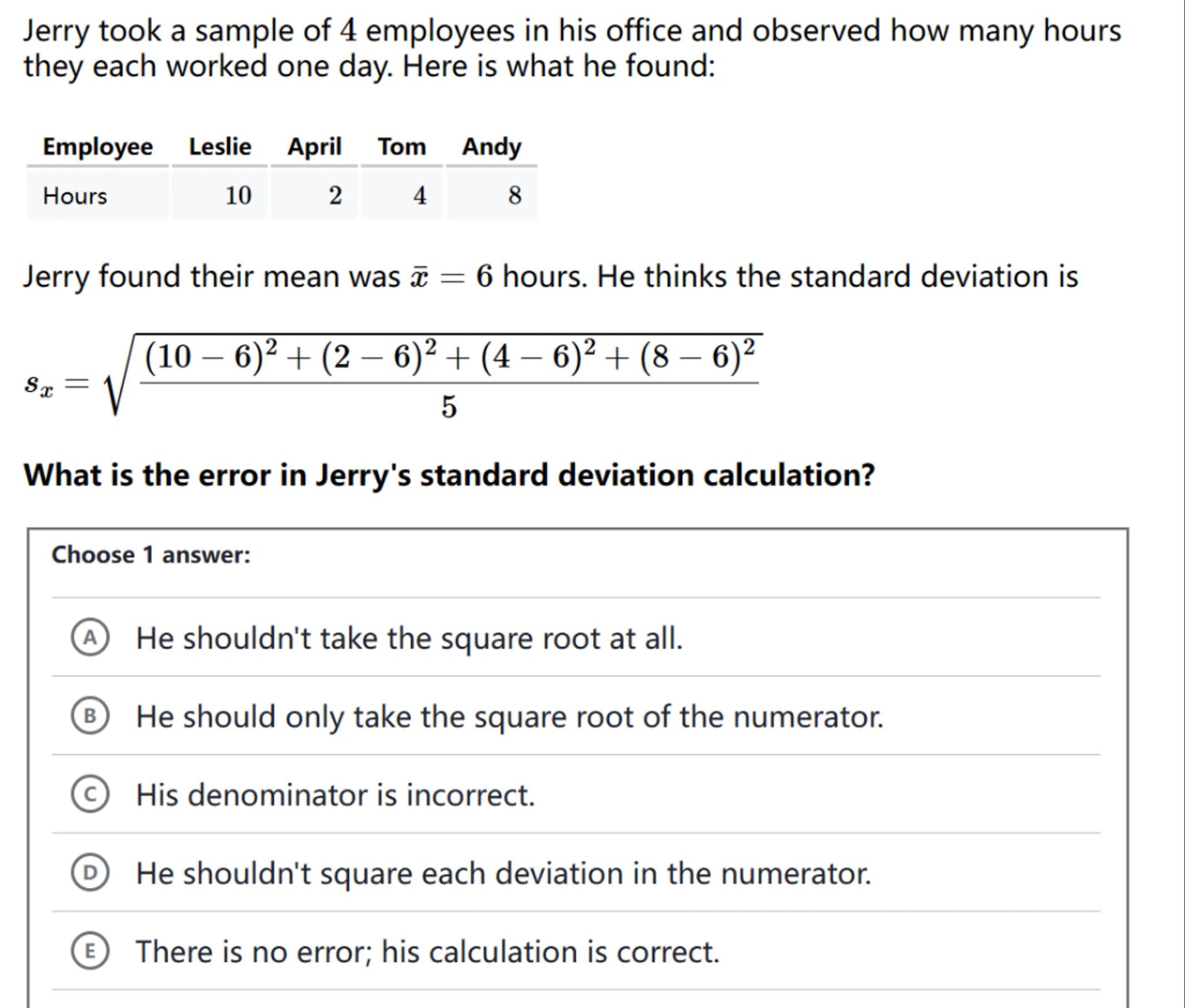

Answer from Sia
Posted over 1 year ago
Solution by Steps
step 2
Note that Jerry used the denominator of 5 instead of the correct denominator , which should be for a sample size of 4
step 3
Correct Jerry's calculation by using the right denominator:
[question number] Answer
C
Key Concept
Sample Standard Deviation
Explanation
The sample standard deviation formula requires dividing by instead of to account for the degrees of freedom in the sample. Jerry used the wrong denominator in his calculation.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question