
Sia

Question
Math
Posted over 1 year ago
Julie is measuring two cones.
Given the base radius and height of the first cone, Julie uses the formula
to compute its volume to be 6 cubic meters.
The second cone has the same height, but has 2 times the radius. What is its volume?
Choose 1 answer:
(A) 12 cubic meters
(B) cubic meters
(C) 24 cubic meters
(D) cubic meters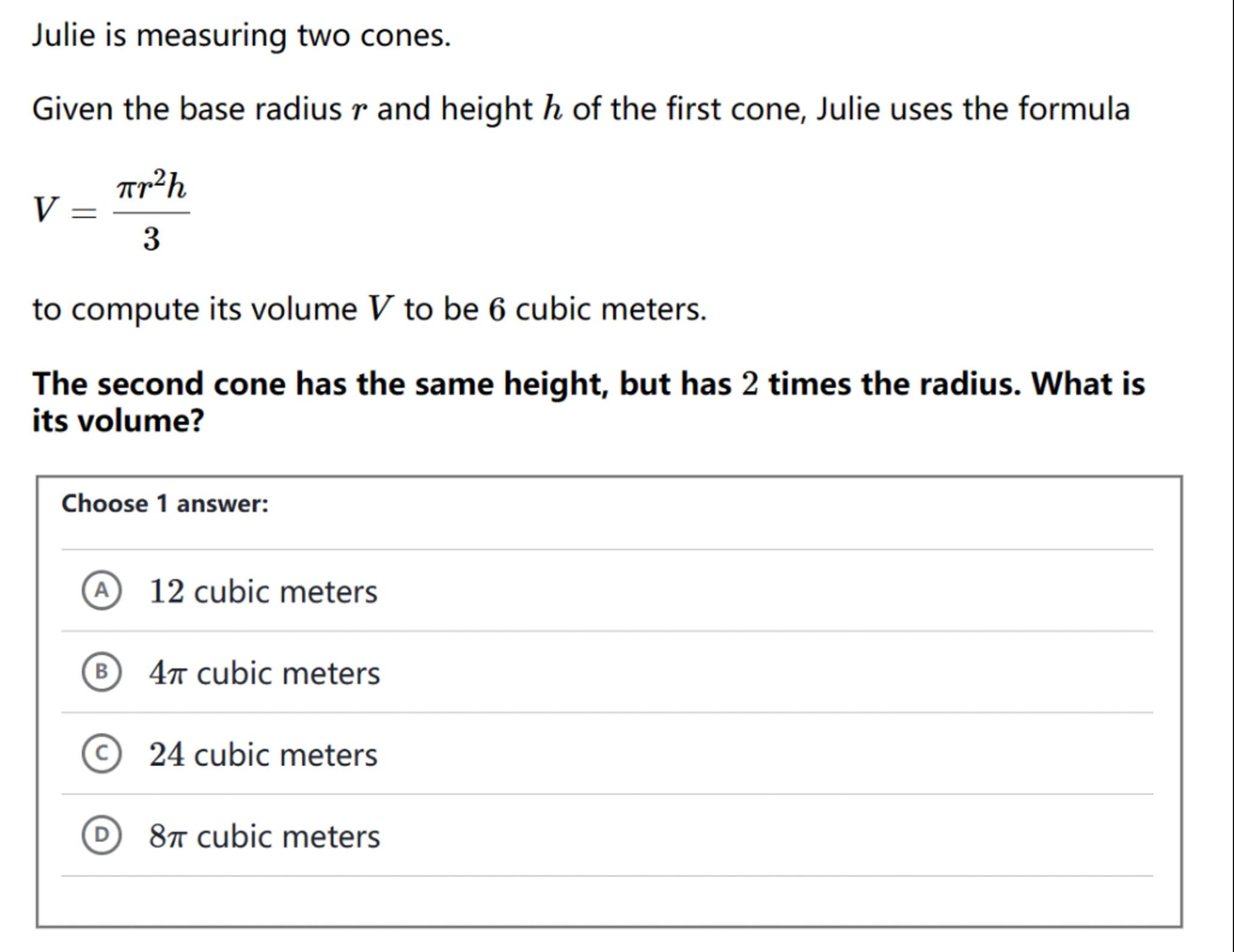

Answer from Sia
Posted over 1 year ago
Solution by Steps
step 1
Given the formula for the volume of a cone and , solve for
step 2
Substitute into the formula to get
step 3
Multiply both sides by to isolate on one side:
step 4
Divide both sides by to solve for : , with the condition that
step 5
For the second cone, the radius is twice that of the first cone, so the new radius is
step 6
Use the volume formula for the second cone with the new radius:
step 7
Substitute from step 4 into the new volume formula:
step 8
Simplify the expression by canceling out and :
step 9
Calculate the new volume: cubic meters
[question 1] Answer
(C) 24 cubic meters
Key Concept
Scaling dimensions in geometric shapes
Explanation
When the radius is doubled, the area of the base is quadrupled because the area is proportional to the square of the radius. Since the height remains the same, the volume is also quadrupled.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question