
Sia

Question
Math
Posted about 1 year ago
Let be the fourth-degree Taylor polynomial for the function about 4 . Assume has derivatives of all orders for all real numbers.
(a) Find and .
(b) Write the second-degree Taylor polynomial for about 4 and use it to approximate .
(c) Write the fourth-degree Taylor polynomial for about 4 .
(d) Can be determined from the information given? Justify your answer.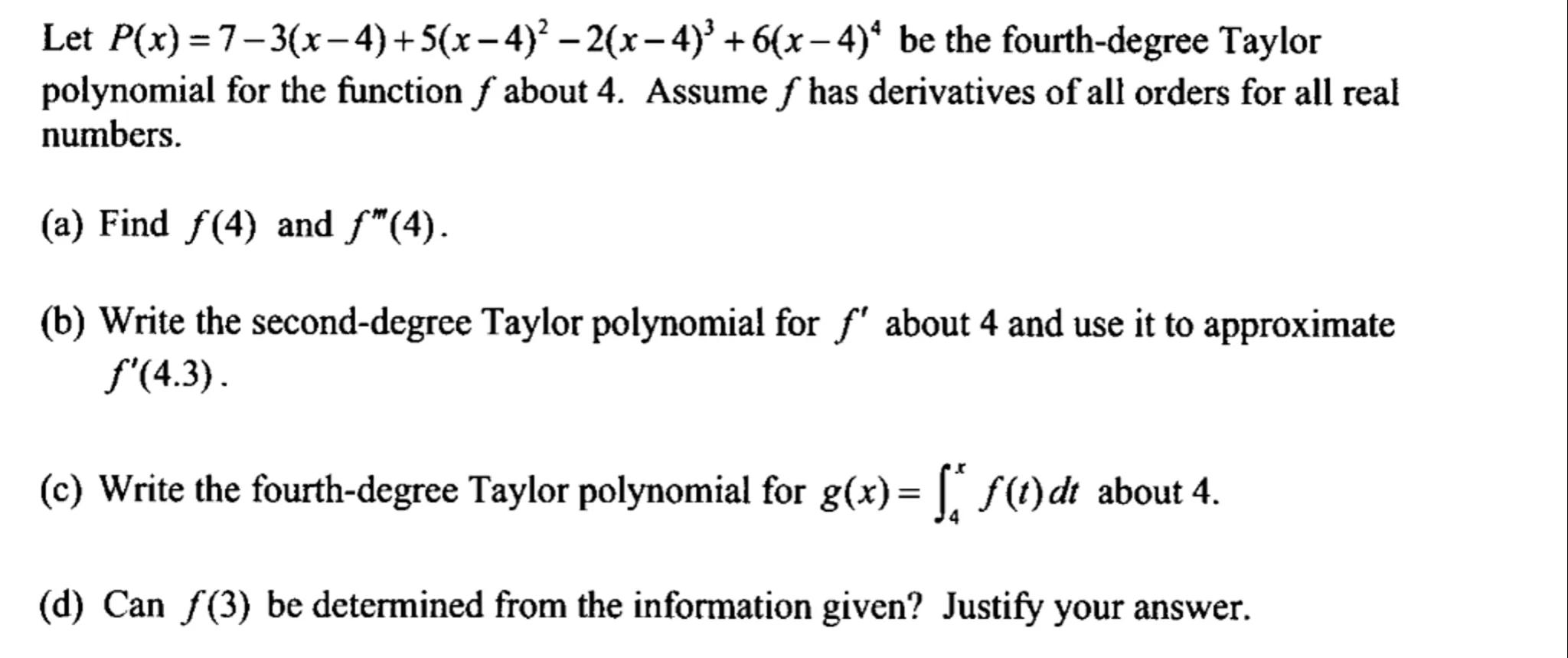

Answer from Sia
Posted about 1 year ago
Solution by Steps
step 1
To find , we evaluate the polynomial at
step 2
step 3
Therefore,
step 4
To find , we need to differentiate twice and evaluate at
step 5
step 6
step 7
step 8
step 9
Therefore,
step 10
To write the second-degree Taylor polynomial for about , we use
step 11
step 12
The second-degree Taylor polynomial for is
step 13
To approximate , we evaluate the second-degree Taylor polynomial at
step 14
step 15
step 16
step 17
Therefore,
step 18
To write the fourth-degree Taylor polynomial for about , we integrate
step 19
step 20
step 21
Therefore, the fourth-degree Taylor polynomial for is
step 22
To determine if can be found from the given information, we note that is centered at
step 23
Since is a Taylor polynomial centered at , it does not provide information about at
step 24
Therefore, cannot be determined from the given information
Answer
, , , fourth-degree Taylor polynomial for is , and cannot be determined.
Key Concept
Taylor Polynomial Approximation
Explanation
Taylor polynomials provide approximations of functions around a specific point, but they do not give information about the function at points far from the center of the polynomial.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question