
Sia

Question
Math
Posted over 1 year ago
Nkechi took a random sample of 10 countries to study fertility rate (babies per woman) and life expectancy (in years). She noticed a strong negative linear relationship between those variables in the sample data. Here is computer output from a least-squares regression analysis for using fertility rate to predict life expectancy:
\begin{tabular}{lrrrrr}
Predictor & Coef & SE Coef & T & P \\
\hline Constant & 89.70 & 1.63 & 54.87 & 0.00 \\
Fertility & -5.97 & 0.59 & -10.18 & 0.00 \\
S & R-sq & & & \\
\hline
\end{tabular}
Use this model to predict the life expectancy of a country whose fertility rate is 2 babies per woman.
You may round your answer to the nearest whole number of years.
years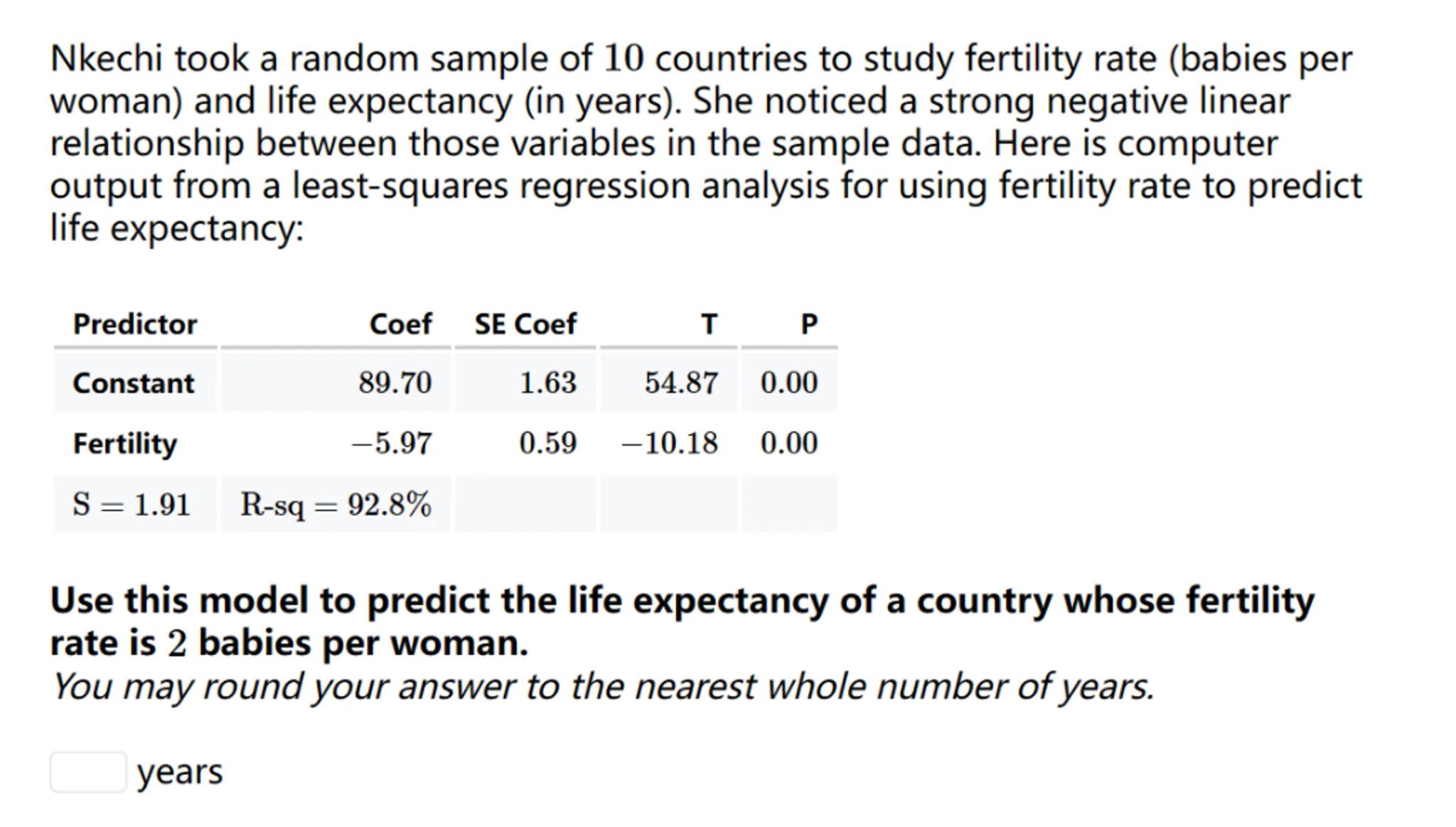

Answer from Sia
Posted over 1 year ago
Solution by Steps
step 1
To predict the life expectancy using the regression model, we use the coefficients for Constant and Fertility
step 2
The regression equation is
step 3
Substitute the given values into the equation:
step 4
Calculate the predicted life expectancy:
step 5
Round the predicted life expectancy to the nearest whole number: years
[1] Answer
78
Key Concept
Linear Regression Prediction
Explanation
To predict a value using a linear regression model, we plug the independent variable(s) into the regression equation and perform the necessary calculations. The result is then rounded to the desired level of precision.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question