
Sia

Question
Math
Posted over 1 year ago
Olivia works at a company that creates mobile phones. She wanted to estimate the mean amount of time their new phone's battery lasts with regular use after a full charge. She took a random sample of 6 of these phones and randomly assigned each of them to a volunteer. She instructed them to fully charge the phones and use them as they regularly would until the battery died (without recharging the phone). Here are the data they reported:
\begin{tabular}{rrrrrrr}
Phone & 1 & 2 & 3 & 4 & 5 & 6 \\
\hline Battery life (hours) & 8.0 & 6.0 & 10.5 & 9.0 & 8.5 & 12 \\
Mean & hours & & & & &
\end{tabular}
Standard deviation hours
Assume that all conditions for inference are met.
Which of the following is a confidence interval for the mean battery life (in hours)?
Choose 1 answer:
(A)
(B)
(C)
(D) 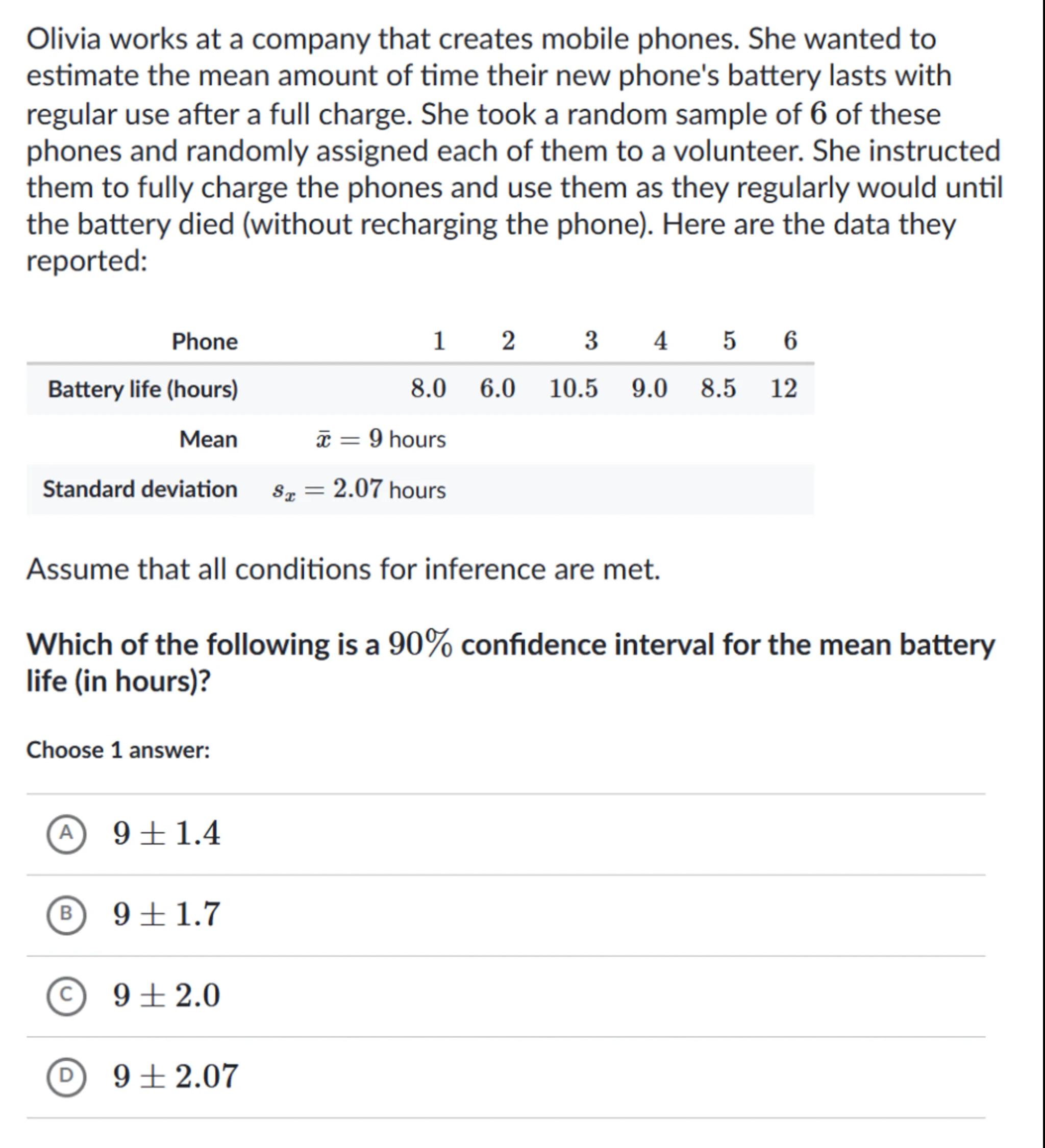

Answer from Sia
Posted over 1 year ago
Solution by Steps
step 2
Given that the sample mean hours, the sample standard deviation hours, the sample size , and the confidence level , we can plug these values into the formula
step 3
The critical value for a 90% confidence interval with 5 degrees of freedom (since ) can be found in a t-distribution table or using a calculator
step 4
The calculation yields a confidence interval of . This matches option (D) from the multiple-choice answers provided
D
Key Concept
T-interval for a population mean
Explanation
The T-interval formula is used to estimate the population mean from a sample mean, standard deviation, and size, with a given confidence level.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question