
Sia
Solve the equations by completing the square.
You will need to work them out on paper.
Give exact answers for these questions:
Give your answers to . or better for these questions:
or
or
or
or
[12]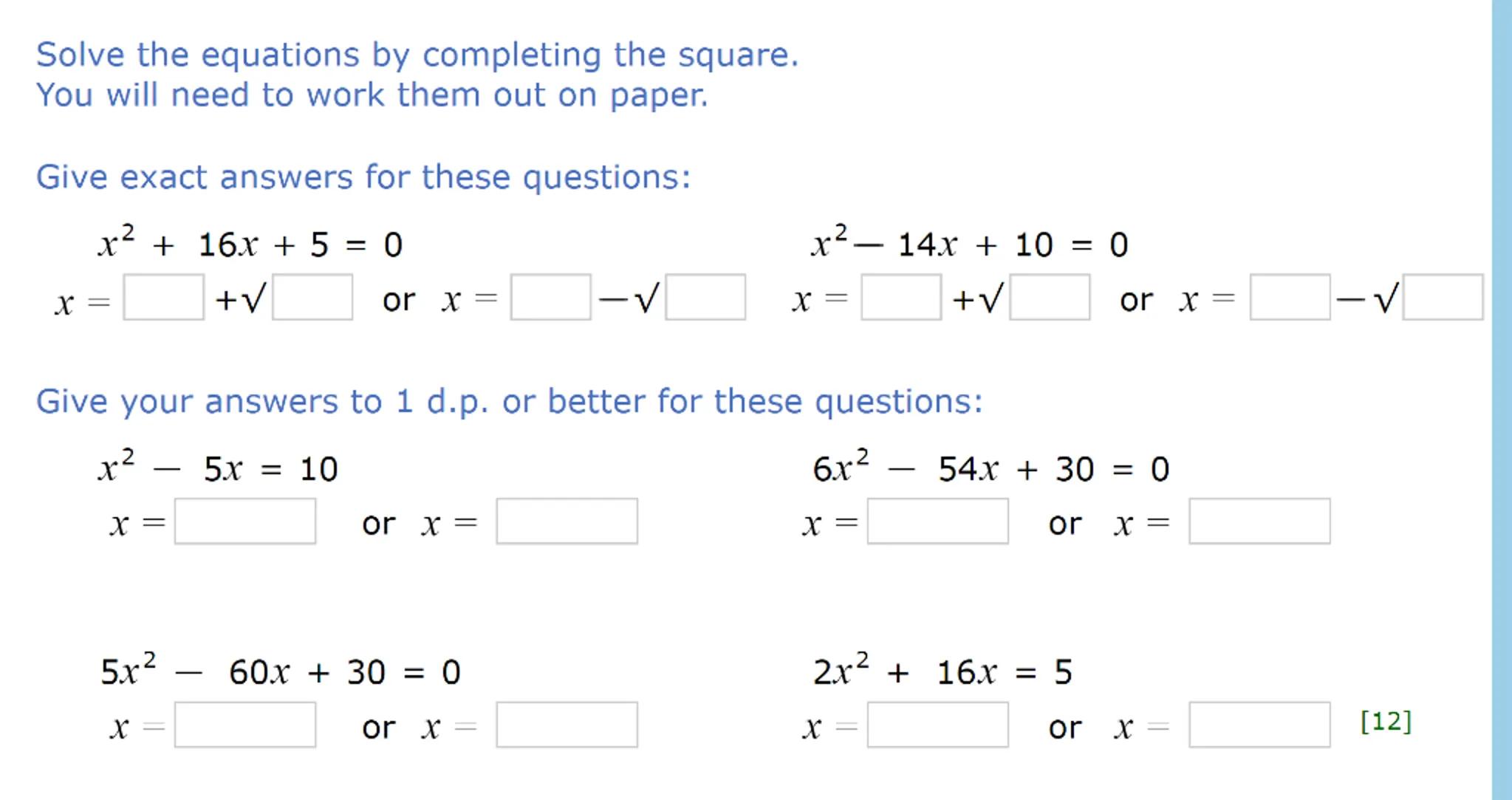

Answer from Sia
Posted about 1 year ago
Solution by Steps
step 1
To solve the equation , we can complete the square. First, we rewrite it as
step 2
Next, we take half of the coefficient of , which is , and square it to get . We add to both sides:
step 3
Now, we can factor the left side: . Taking the square root of both sides gives us
step 4
Finally, we solve for :
step 5
For the second equation , we rewrite it as
step 6
Taking half of the coefficient of , which is , and squaring it gives us . We add to both sides:
step 7
Factoring gives us . Taking the square root of both sides gives us
step 8
Finally, we solve for :
step 9
For the equation , we rewrite it as
step 10
Completing the square gives us
step 11
Factoring gives us . Taking the square root gives us
step 12
Finally, we solve for :
step 13
For the equation , we divide by 6 to simplify:
step 14
Completing the square gives us
step 15
Factoring gives us . Taking the square root gives us
step 16
Finally, we solve for :
step 17
For the equation , we divide by 5 to simplify:
step 18
Completing the square gives us
step 19
Factoring gives us . Taking the square root gives us
step 20
Finally, we solve for :
step 21
For the equation , we rewrite it as
step 22
Completing the square gives us
step 23
Factoring gives us . Taking the square root gives us
step 24
Finally, we solve for :
Answer
For the equations:
1.
2.
3.
4.
5.
6.
Key Concept
Completing the square is a method used to solve quadratic equations by rewriting them in a perfect square form.
Explanation
The solutions are derived by transforming the quadratic equations into a form that allows us to easily extract the roots. Each step involves manipulating the equation to isolate the variable.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question
