
Sia

Question
Math
Posted over 1 year ago
The Department of Tourism reported that in May of 2017, approximately of the tourists to the Philippines were from Asia. They also noted that it was the first time that the Philippines had more than 500,000 tourists in the month of May. Suppose another organization had taken a simple random sample of 600 of the tourists in that population.
Assuming that the reported claim is accurate, what is the approximate probability that the other organization's results were within 2 percentage points of the Department of Tourism's results?
Choose 1 answer:
(A)
(B)
(C)
(D) 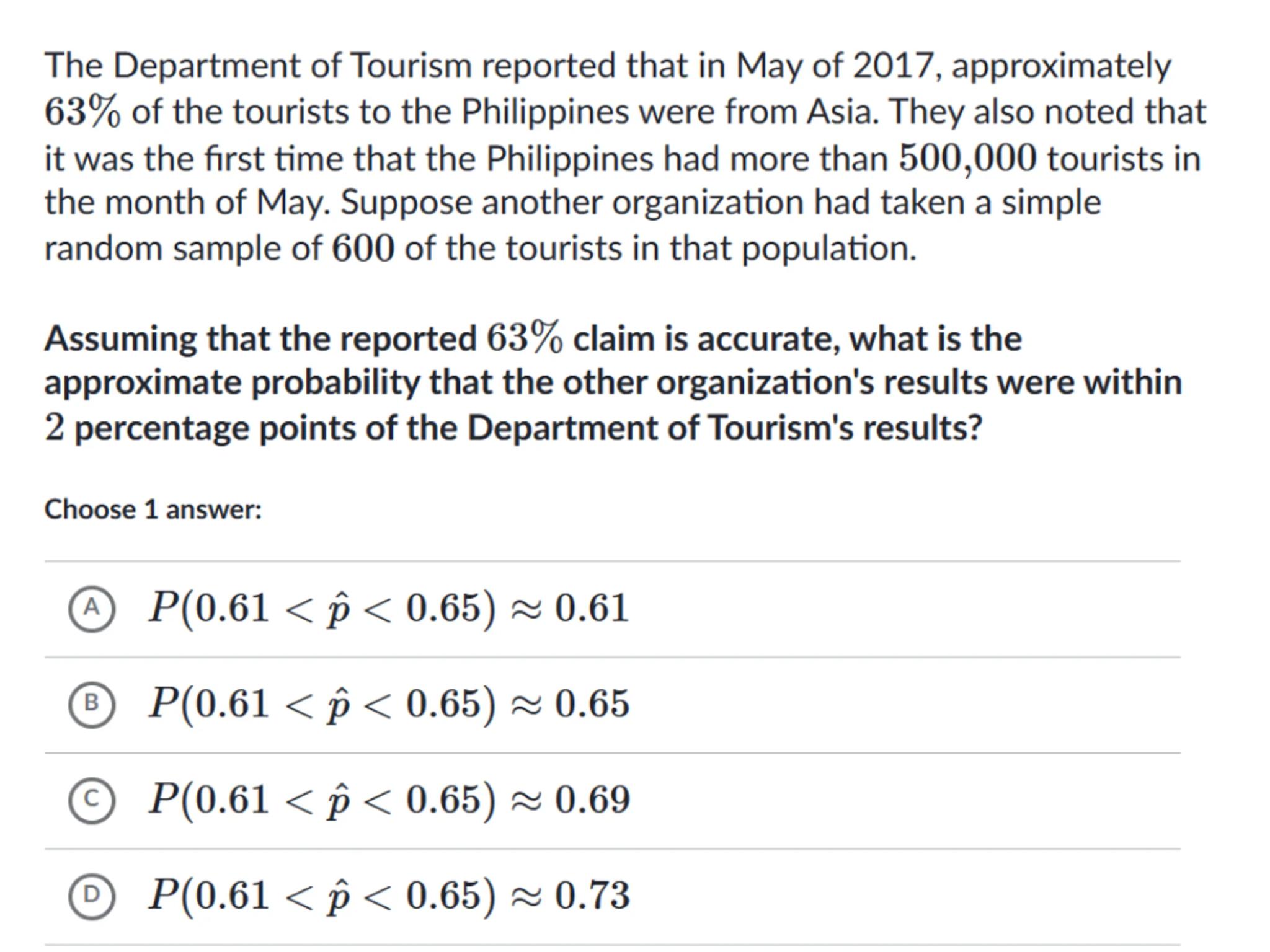

Answer from Sia
Posted over 1 year ago
Solution by Steps
step 2
Substitute the given values into the formula to get
step 3
Compute the standard deviation:
step 4
Convert the range of proportions (0.61 to 0.65) into z-scores by subtracting the mean (0.63) and dividing by the standard deviation ()
step 5
Calculate the z-score for 0.61:
step 6
Calculate the z-score for 0.65:
step 7
Use the standard normal distribution table to find the probabilities corresponding to the z-scores
step 8
The probability for is approximately 0.1611, and for is approximately 0.8389
step 9
Subtract the probability for from the probability for to find the probability that the sample proportion is between 0.61 and 0.65:
step 10
The closest answer choice to 0.6778 is 0.69
C
Key Concept
Normal Approximation to the Binomial Distribution
Explanation
When the sample size is large, the sampling distribution of the sample proportion can be approximated by a normal distribution with mean equal to the population proportion and standard deviation calculated using the formula . The z-scores are then used to find the probabilities for a given range.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question