
Sia
The circuit shown in the figure employs an operational amplifier that can be considered ideal. Determine the value of the current delivered to the load resistance .
Data: .
(Glossary: volt, meter, ampere, watt, ohm, farad, hertz, second)
Select one:
a.
b.
c.
d. 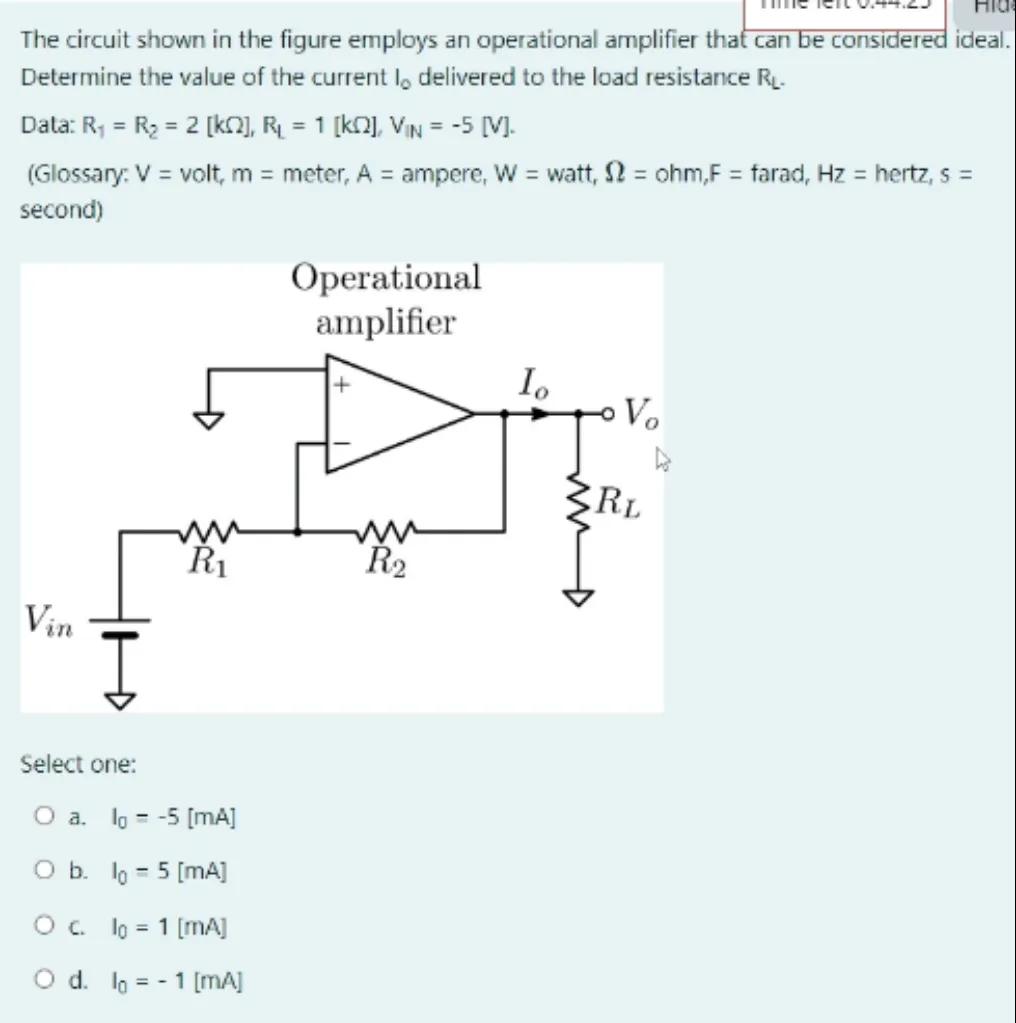

Answer from Sia
Posted over 1 year ago
D
Key Concept
Inverting Operational Amplifier
Explanation
In an inverting operational amplifier, the output voltage is given by . Given and , the output voltage . The current through the load resistor is . Since the current direction is opposite to the assumed direction, .
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question
