
Sia

Question
Math
Posted over 1 year ago
The function models the average high temperature, in degrees Celsius, of Paityn's town on the day of the year. The function models the average high temperature of Vince's town on the day of the year.
Consider the following equation:
What does the solution set for the last equation represent?
Choose 1 answer:
(A) The maximum average high temperature in Paityn's town
(B) The maximum average high temperature in Vince's town
(C) The number of days per year when the towns have the same average high temperature
(D) The set of all days when the towns have the same average high temperature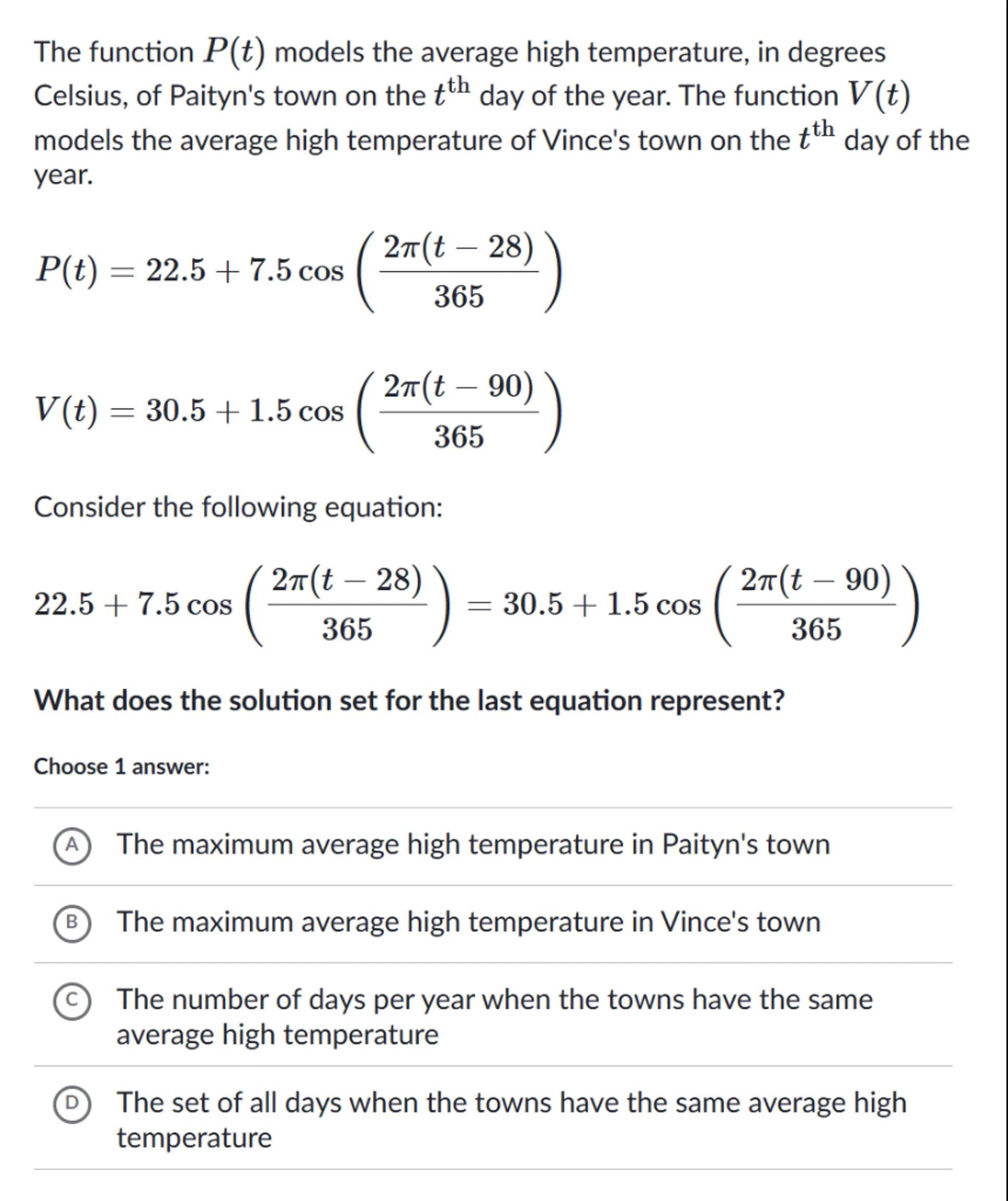

Answer from Sia
Posted over 1 year ago
Solution by Steps
step 2
According to the asksia-ll calculation list, the solutions to the equation are and where is an element of the set of integers . This indicates that there are multiple solutions to the equation, corresponding to different days of the year
step 3
Since the solutions represent multiple days of the year, the solution set of the equation represents all the days when the average high temperatures in Payton's town and Vince's town are the same
D
Key Concept
Solution Set Interpretation
Explanation
The solution set of the equation represents all the days of the year when the average high temperatures in Payton's town and Vince's town are equal.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question