
Sia

Question
Math
Posted over 1 year ago
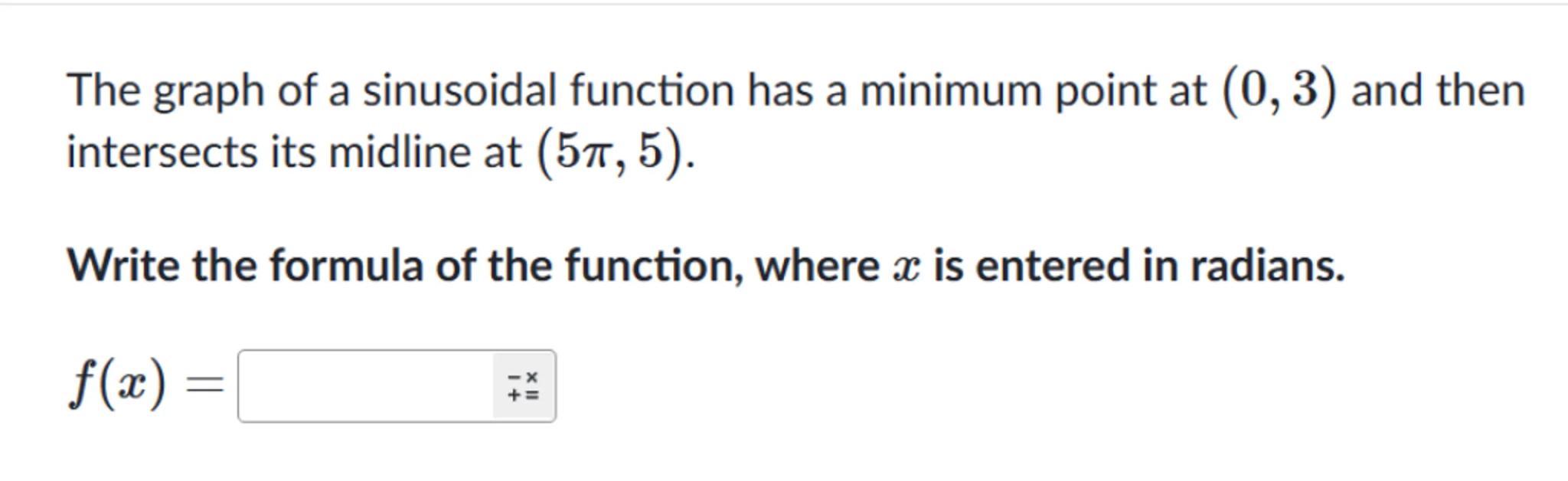
The graph of a sinusoidal function has a minimum point at and then intersects its midline at .
Write the formula of the function, where is entered in radians.

Answer from Sia
Posted over 1 year ago
Solution by Steps
step 1
Identify the characteristics of the sinusoidal function from the given points
step 2
The minimum point at indicates that the phase shift is and the vertical shift is
step 3
The function intersects its midline at , which is one period away from the minimum. This tells us the midline is and the amplitude is
step 4
Since the period is , we can find the angular frequency, , using the formula
step 5
Calculate the angular frequency:
step 6
Write the formula of the sinusoidal function using the amplitude , angular frequency , phase shift , and vertical shift :
step 7
Substitute the known values into the formula:
Answer
Key Concept
Writing the formula of a sinusoidal function
Explanation
The formula of a sinusoidal function is derived from its amplitude, period (which gives the angular frequency), phase shift, and vertical shift. These values are determined from the characteristics of the function as described by its graph or given points.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question