
Sia

Question
Math
Posted about 1 year ago
\begin{tabular}{|c||c|c|c|c|}
\hline & & & & \\
\hline 0 & 3 & -2 & 1 & 4 \\
\hline 1 & 2 & -3 & 3 & -2 \\
\hline 2 & -1 & 1 & 4 & 5 \\
\hline
\end{tabular}
5. Selected values of a function and its first three derivatives are indicated in the table above. What is the third-degree Taylor polynomial for about ?
(A)
(B)
(C)
(D) 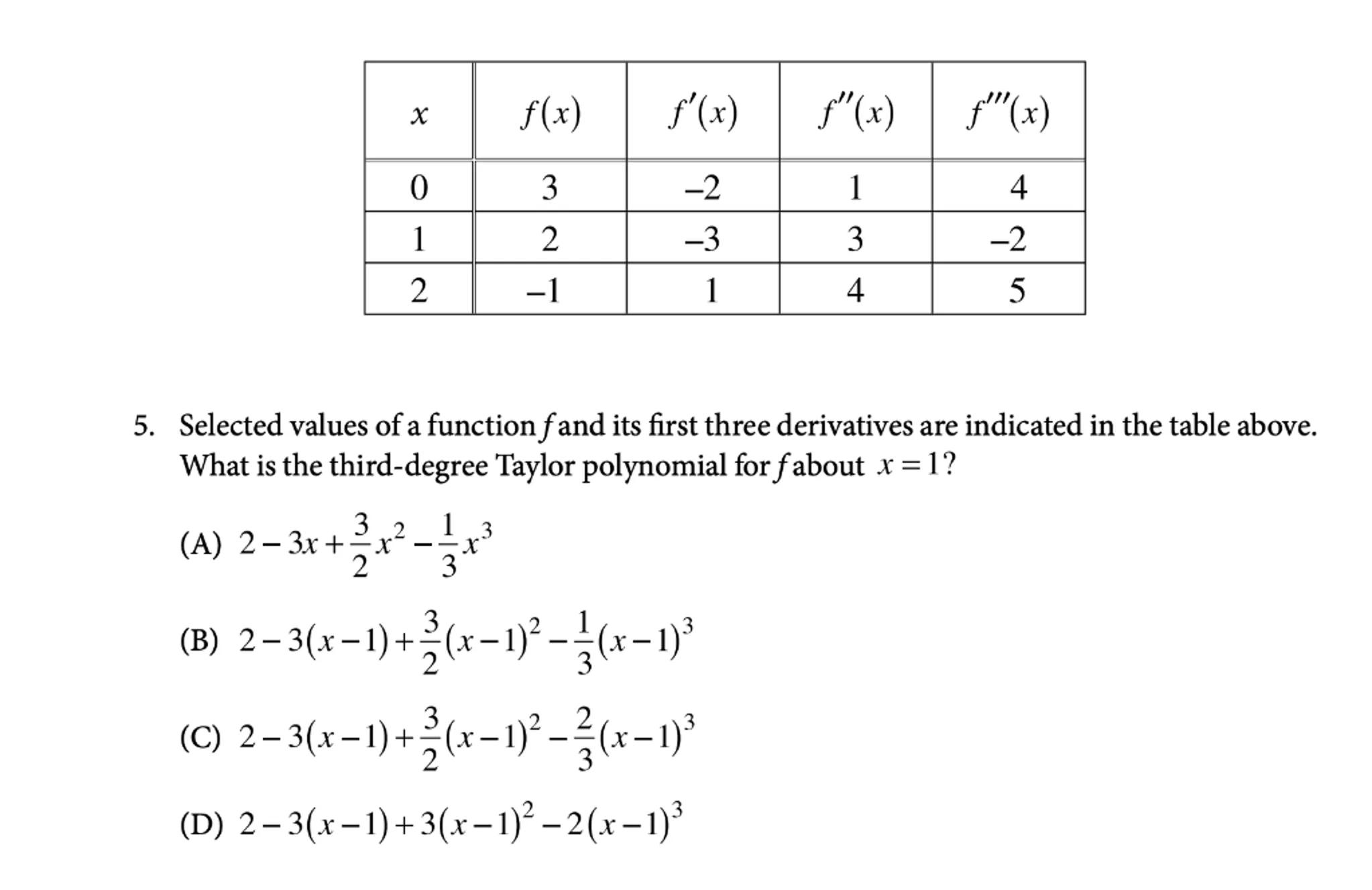

Answer from Sia
Posted about 1 year ago
Solution by Steps
step 2
From the table, we have:
step 3
Substituting these values into the Taylor polynomial formula, we get:
B
Key Concept
Taylor Polynomial
Explanation
The Taylor polynomial of degree for a function about is given by .
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question