
Sia

Question
Chemistry
Posted over 1 year ago
2. In a vacuum chamber of , the air is heated to . Determine convertion ratio of NO at chemical equilibrium state?
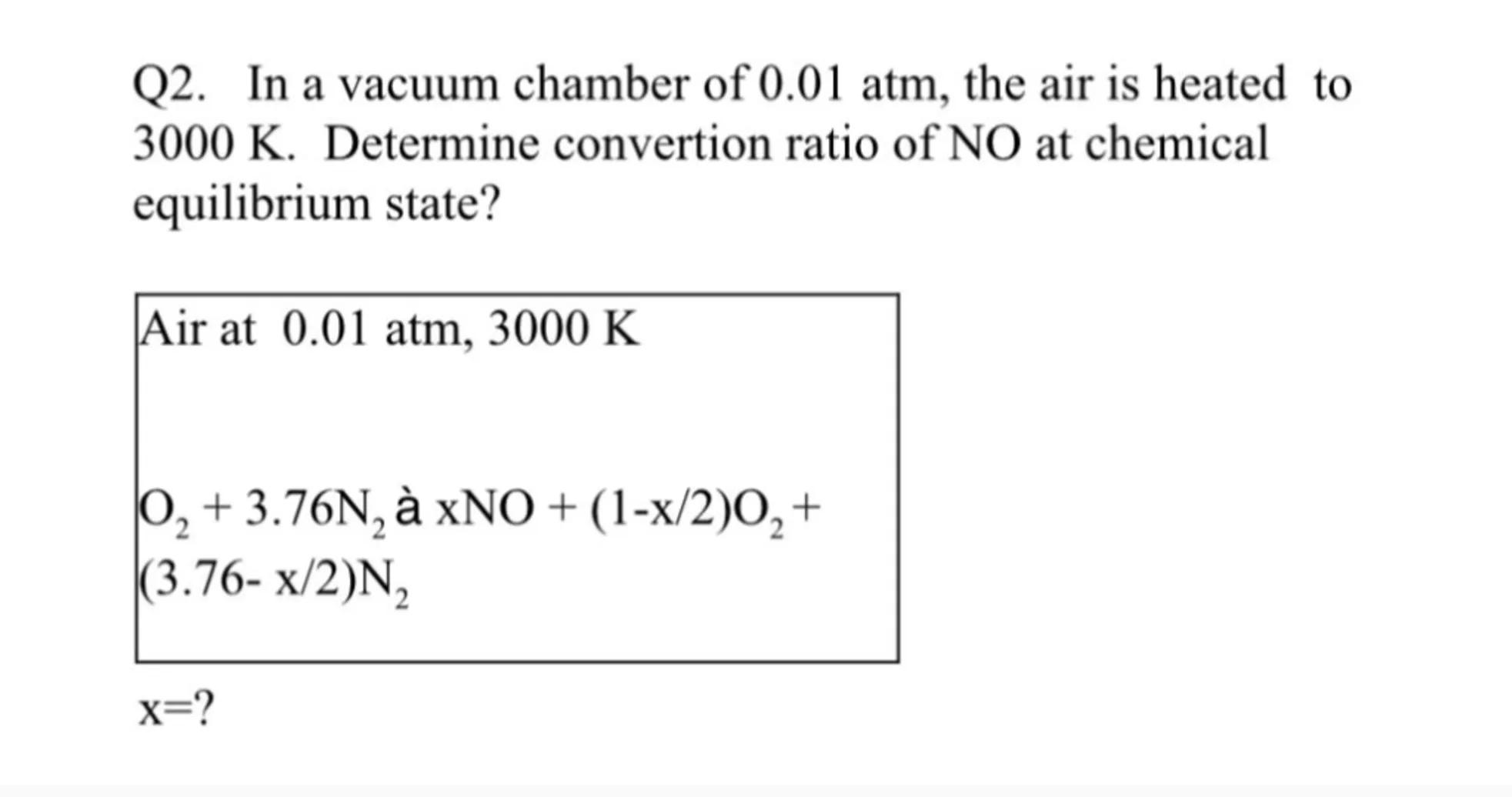

Answer from Sia
Posted over 1 year ago
Solution
1
Identify the reaction: The given reaction is the formation of NO from O and N in air at 0.01 atm and 3000 K. The reaction can be written as:
2
Determine the equilibrium constant (K): At high temperatures, the equilibrium constant for the formation of NO can be approximated using the van't Hoff equation. For simplicity, we assume the equilibrium constant is known or can be found in tables for the given temperature (3000 K)
3
Set up the equilibrium expression: The equilibrium constant expression for the reaction is:
Given the partial pressures at equilibrium, we can express the concentrations in terms of :
where is the volume of the chamber
4
Solve for x: Substitute these expressions into the equilibrium constant equation and solve for :
Simplify and solve for :
This equation can be solved numerically to find the value of
Answer
The conversion ratio of NO at chemical equilibrium state is determined by solving the equilibrium constant expression for .
Key Concept
The equilibrium constant expression relates the concentrations of reactants and products at equilibrium.
Explanation
By setting up the equilibrium constant expression and solving for the unknown , we can determine the conversion ratio of NO in the given conditions.
Not the question you are looking for? Ask here!
Enter question by text
Enter question by image
Unlock Smarter Learning with AskSia Super!
Join Super, our all-in-one AI solution that can greatly improve your learning efficiency.
30% higher accuracy than GPT-4o
Entire learning journey support
The most student-friendly features
Study Other Question